- #1
giraffe714
- 5
- 2
- Homework Statement
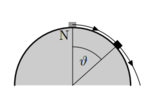
- A point mass is placed at the north pole of a ball within a homogeneous vertical gravitational field with acceleration g. The mass therefore resides in an unstable equilibrium
from which it is removed by a negligibly small kick. It then glides without friction down
the surface of the ball. At which angle θ does the mass lift off from the surface of the
ball? Hint: To obtain the velocity of the mass as a function of the angle, you can use conservation
of energy. Alternatively, you can multiply the equation of motion for θ(t) by ##\dot{\theta}## and
integrate over time.
- Relevant Equations
- ##\frac{d}{dt}\frac{\partial L}{\partial \dot{\theta}} = \frac{\partial L}{\partial \theta}##, L = T - V
[Rewriting this as per the suggestions. Thanks once again.]
I won't be using the Lagrangian because it was never explicitly stated that I have to so I'll just use conservation of energy.
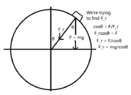
$$ T = \frac{1}{2}mv^2 = \frac{1}{2}m(R\dot{\theta})^2 = \frac{1}{2}mR^2\dot{\theta}^2 $$
$$ V = mgy = mgRcos\theta $$
At the topmost point, just when the mass is being pushed, the kinetic energy is 0 because the velocity is 0. At the topmost point since ##\theta## is 0 ##cos\theta## = 1, therefore ##V = mgR##
Since T + V is constant, we can write this as
$$ \frac{1}{2}mR^2\dot{\theta}^2 + mgRcos\theta = mgR $$
The units of the kinetic energy are kg*m^2*rad^2/s^2, but since radians aren't technically units this just becomes kg*m^2/s^2 or J. ##mgRcos\theta## also holds up, kg*m/s^2*m or once again J. Same thing with the right hand side.
Now we can cancel out m's and R's:
$$ \frac{1}{2}R\dot{\theta}^2 + gcos\theta = g $$
Which then gives that
$$ \frac{1}{2}R\dot{\theta}^2 = g - gcos\theta \rightarrow \dot{\theta}^2 = \frac{2g - 2gcos\theta}{R} \rightarrow \dot{\theta} = \sqrt{\frac{2g - 2gcos\theta}{R}} $$
Is this dimensionally consistent? On the left side we have rad/s (or just 1/s), and on the right side we have... ## \sqrt{\frac{m/s^2}{m}} = \sqrt{1/s^2} = 1/s ## Yes, that checks out so far.
Now for the constraint condition: F_r = F_c. ## F_r = mg/cos\theta ## (once again from diagram) and ## F_c = \frac{mv^2}{R} ##, the latter of which is also from my textbook so I would really hope it's dimensionally consistent . Also, ##v = R\dot{\theta}##.
. Also, ##v = R\dot{\theta}##.
$$ \frac{mg}{cos\theta} = \frac{mv^2}{R} \rightarrow \frac{mg}{cos\theta} = \frac{mR^2\dot{\theta}^2}{R} = mR\dot{\theta}^2 \rightarrow \frac{g}{cos\theta} = R\dot{\theta}^2 $$
This is certainly cleaner than whatever I wrote down last time so I'm hoping this actually works out. As for the units, the left side has units of m/s^2 and the right has units of 1/s^2*m. I'm just ignoring the radians because they're dimensionless units and don't affect this analysis.
$$ \frac{g}{cos\theta} = R\frac{2g - 2gcos\theta}{R} \rightarrow cos\theta = 2 - 2cos\theta $$
$$ 1 = \frac{2}{cos\theta} - 2 \rightarrow 3 = \frac{2}{cos\theta} \rightarrow cos\theta = \frac{2}{3} $$
$$ \theta = arccos\frac{2}{3} $$
And even the units check out, so, I guess I solved it? If there are still any errors do point them out but I actually think I did get everything this time. Thanks for the help folks!
I won't be using the Lagrangian because it was never explicitly stated that I have to so I'll just use conservation of energy.
$$ T = \frac{1}{2}mv^2 = \frac{1}{2}m(R\dot{\theta})^2 = \frac{1}{2}mR^2\dot{\theta}^2 $$
$$ V = mgy = mgRcos\theta $$
At the topmost point, just when the mass is being pushed, the kinetic energy is 0 because the velocity is 0. At the topmost point since ##\theta## is 0 ##cos\theta## = 1, therefore ##V = mgR##
Since T + V is constant, we can write this as
$$ \frac{1}{2}mR^2\dot{\theta}^2 + mgRcos\theta = mgR $$
The units of the kinetic energy are kg*m^2*rad^2/s^2, but since radians aren't technically units this just becomes kg*m^2/s^2 or J. ##mgRcos\theta## also holds up, kg*m/s^2*m or once again J. Same thing with the right hand side.
Now we can cancel out m's and R's:
$$ \frac{1}{2}R\dot{\theta}^2 + gcos\theta = g $$
Which then gives that
$$ \frac{1}{2}R\dot{\theta}^2 = g - gcos\theta \rightarrow \dot{\theta}^2 = \frac{2g - 2gcos\theta}{R} \rightarrow \dot{\theta} = \sqrt{\frac{2g - 2gcos\theta}{R}} $$
Is this dimensionally consistent? On the left side we have rad/s (or just 1/s), and on the right side we have... ## \sqrt{\frac{m/s^2}{m}} = \sqrt{1/s^2} = 1/s ## Yes, that checks out so far.
Now for the constraint condition: F_r = F_c. ## F_r = mg/cos\theta ## (once again from diagram) and ## F_c = \frac{mv^2}{R} ##, the latter of which is also from my textbook so I would really hope it's dimensionally consistent
$$ \frac{mg}{cos\theta} = \frac{mv^2}{R} \rightarrow \frac{mg}{cos\theta} = \frac{mR^2\dot{\theta}^2}{R} = mR\dot{\theta}^2 \rightarrow \frac{g}{cos\theta} = R\dot{\theta}^2 $$
This is certainly cleaner than whatever I wrote down last time so I'm hoping this actually works out. As for the units, the left side has units of m/s^2 and the right has units of 1/s^2*m. I'm just ignoring the radians because they're dimensionless units and don't affect this analysis.
$$ \frac{g}{cos\theta} = R\frac{2g - 2gcos\theta}{R} \rightarrow cos\theta = 2 - 2cos\theta $$
$$ 1 = \frac{2}{cos\theta} - 2 \rightarrow 3 = \frac{2}{cos\theta} \rightarrow cos\theta = \frac{2}{3} $$
$$ \theta = arccos\frac{2}{3} $$
And even the units check out, so, I guess I solved it? If there are still any errors do point them out but I actually think I did get everything this time. Thanks for the help folks!
Attachments
Last edited: