A Physics Misconception with Gauss’ Law

Introduction

It is relatively common to see the following type of argument:

The surface area is ##A## and the enclosed charge is ##Q##. The electric field strength on the surface is therefore ##E = Q/\varepsilon_0 A##.

The problem is that this statement is only true in very special cases. In this Insight, we discuss the underlying assumptions required and what Gauss’ law actually says.

What Gauss’ law says

Gauss’ law on integral form relates the flux of the electric field through a closed surface to the charge enclosed by the surface ##\varepsilon_0 \Phi = Q_{\rm enc}##. Here ##\Phi## is the flux, ##Q_{\rm enc}## the enclosed charge, and ##\varepsilon_0## the permittivity of vacuum. In slightly more mathematical terms, $$\varepsilon_0 \oint_S \vec E \cdot d\vec S = \int_V \rho \, dV,$$ where ##S## is the surface, ##V## the enclosed volume, and ##\rho## the charge density.

It is important to note that the flux integral only depends on the component of ##\vec E## orthogonal to the surface. Any component parallel to the surface will not lead to a flux out of the surface, see the figure below.

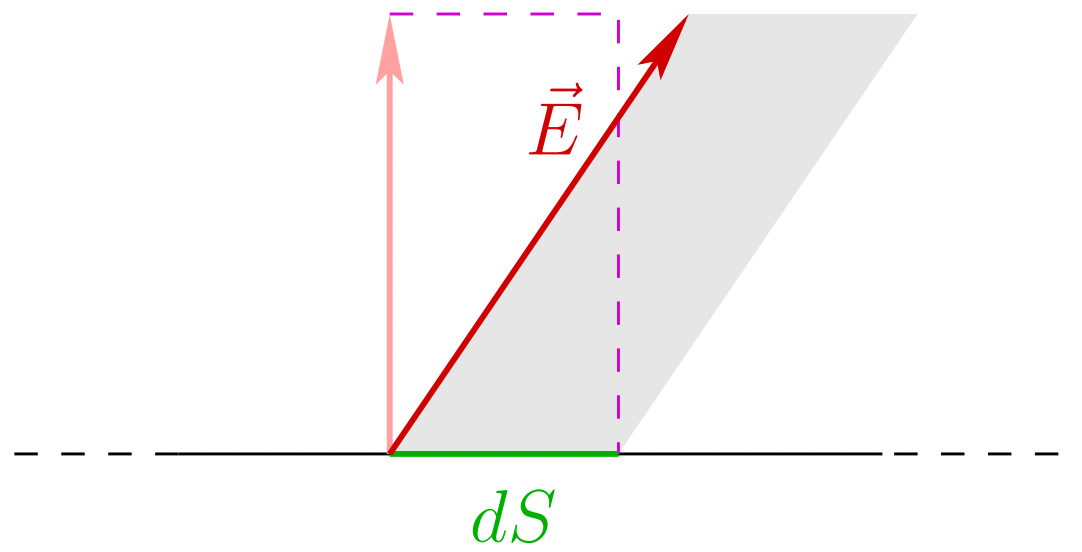
The flux of the electric field ##\vec E## through a small surface element ##dS## is the volume spanned by ##\vec E## and ##dS##. Only the component of ##\vec E## perpendicular to the surface (in pink) is relevant as the gray region has the same volume as that delimited by the purple dashes. Any component parallel to ##dS## does not contribute to the flux.

Mathematically, if ##\vec E## is orthogonal to and of constant magnitude ##E_0## on the surface, then ##\vec E \cdot d\vec S = E_0 dS##. In that case $$\Phi = \oint_S E_0 dS = E_0 \oint_S dS = E_0 A,$$ where ##A## is the area of ##S##. The argument in the introduction is therefore reliant on these two assumptions.

Spherical symmetry

Does the argument work in the case of spherically symmetric charge distribution? Well … Yes and no. The argument does give the correct result but it does not tell the whole story. The spherical symmetry implies that the electric field must be in the form $$\vec E = E(r) \vec e_r.$$ Using a sphere of radius ##R## as our surface ##S##, ##\vec E## is orthogonal to the surface everywhere. Since the area of the sphere is ##A = 4\pi R^2##, it follows that ##\Phi = E(R) A = 4\pi E(R) R^2##. We can therefore express the electric field on the sphere as $$\vec E = \frac{Q_{\rm enc}}{4\pi \varepsilon_0 R^2}.$$

So far, everything looks perfectly fine. However, there are a few key points to this that are missed by the argument at the beginning of the Insight:

• We needed to use a symmetry to conclude the functional form of the electric field.
• From the symmetry, we could also conclude that the field strength was the same on our entire surface. It was crucial to pick a surface that respected the symmetry.
• The symmetry also implied that the field was orthogonal to the surface everywhere.

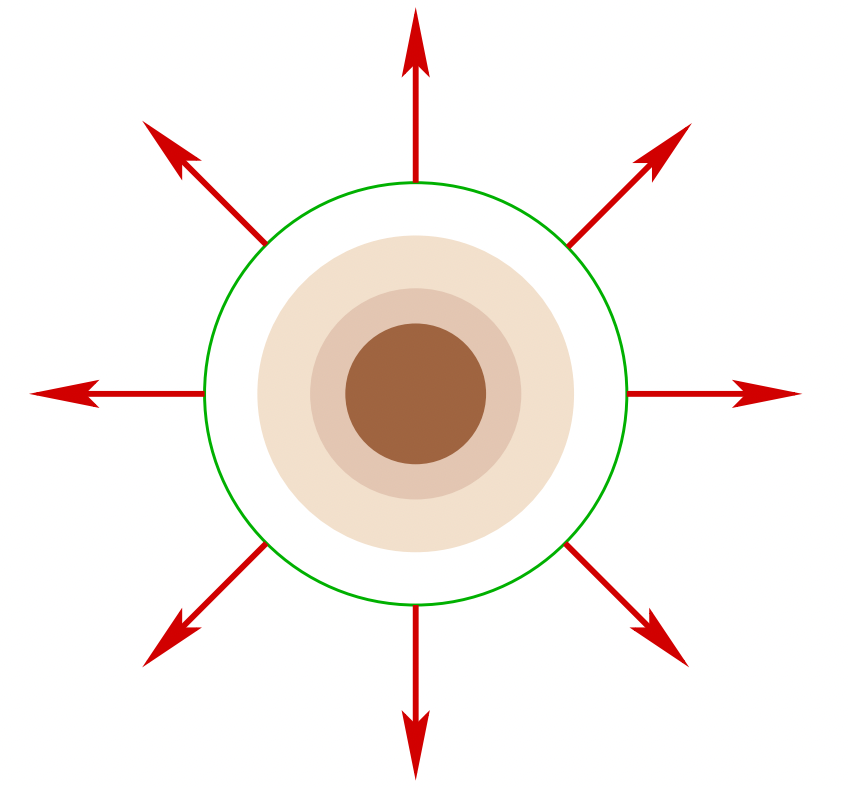
In a spherically symmetric setting, the electric field is always perpendicular to a spherical surface respecting the symmetry. It is also of constant magnitude on the surface.

Because of these three points, we could indeed conclude that ##E = Q/\varepsilon_0 A##. However, each point was necessary to draw this conclusion.

Failure of the argument for spherical symmetry

The conclusion ##E = Q/\varepsilon_0 A## would fail for spherical symmetry if we would have chosen any other surface, see the figure below.

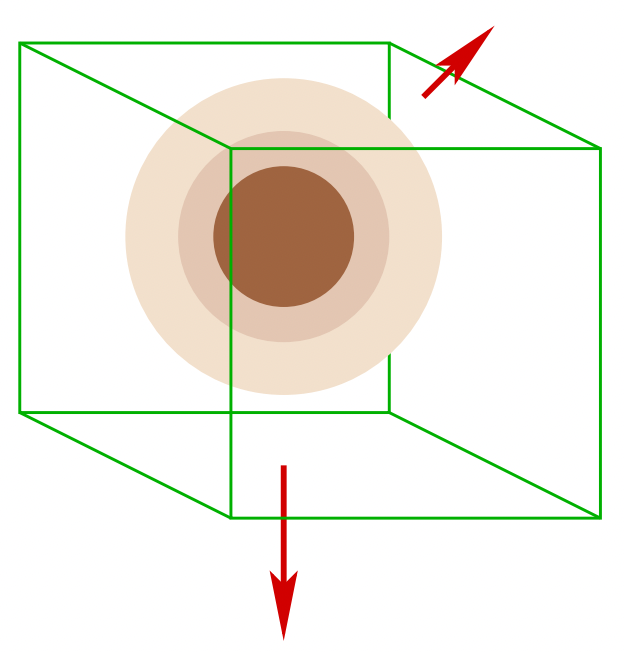
A surface (green) that does not respect the spherical symmetry. The field is neither of constant magnitude nor perpendicular to the surface.

For example, if we had taken a cube instead of a sphere, the electric field would:

• Not have constant magnitude on the surface.
• Not be orthogonal to the surface everywhere.

The conclusion at the beginning of the Insight would conclude that ##E = Q_{\rm enc}/6\varepsilon_0 L^2##, where ##L## is the cube’s side length, which would be incorrect.

Cylinder symmetry

Students often fail to recreate the argument in the case of cylinder symmetry. In particular, this is done for a line charge of linear charge density ##\rho_\ell##. The argument typically takes the following form:

Due to the cylinder symmetry, choose a cylinder surface of radius ##R## and length ##L##, see the figure below.

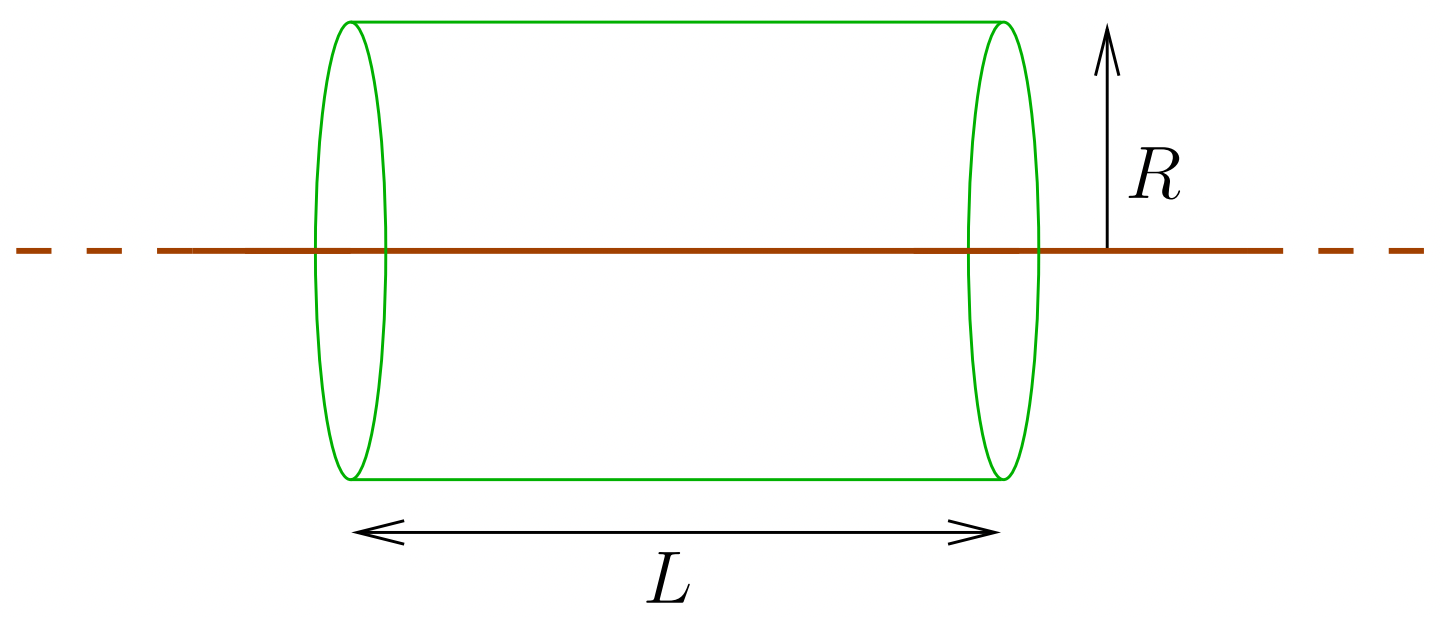
A cylinder (green) surface surrounding an infinite line charge (brown).

The area of the cylinder is the sum of the side area and the base areas ##A = 2\pi RL + 2\pi R^2 = 2\pi R(L+R)##. The charge enclosed by the surface is ##Q_{\rm enc} = \rho_\ell L##. The electric field is therefore $$E = \frac{\rho_\ell L}{2\pi R(L+R)}.$$

This result inherently smells a bit bad. We should not expect the field strength to depend on the length of the cylinder we chose. The dependence on ##R## is however perfectly fine as symmetry arguments would imply that ##\vec E = E(\rho) \vec e_\rho##. Here ##\rho## is the radial coordinate in cylinder coordinates and ##\vec e_\rho## is the corresponding basis vector.

So where does the argument fail? On the side of the cylinder, the field can indeed be argued to be both orthogonal to the surface and of constant magnitude. The flux through the side is therefore ##\Phi_{\rm side} = E(R) 2\pi RL##. On the end caps, the field is parallel to the surface so the flux is ##\Phi_{\rm caps} = 0##. Consequently, the total flux is $$\Phi = E(R) 2\pi RL$$. Since the enclosed charge is ##Q_{\rm enc} = \rho_\ell L##, this implies $$E(R) = \frac{\rho_\ell L}{2\pi \varepsilon_0 RL} = \frac{\rho_\ell}{2\pi \varepsilon_0 R}.$$

Be careful!

In conclusion, be very careful when applying ##E = Q_{\rm enc}/\varepsilon_0 A##. The requirements for this being correct are:

• The area should be that of a surface on which the electric field is orthogonal to the surface.
• The electric field should have a constant magnitude in that area.
• The flux through any other surfaces involved in making a closed surface should be zero.