Frequencies for harmonic oscillator with multiple periodic solutions?

The Fourier series for ##f(t)## is

$$f(t)=\frac{\pi}{2}-\frac{4}{\pi}\sum\limits_{n=1}^\infty \frac{\cos{(n\omega t)}}{n^2}\tag{3}$$

The steady-state periodic solution to the differential equation in ##x## is

$$x_p(t)=\frac{\pi}{2\omega_n^2}-\frac{4}{\pi}\sum\limits_{n=1}^\infty \frac{\cos{(n\omega t)}}{n^2(\omega_n^2-n^2\omega^2)}\tag{4}$$

The derivation of ##(4)## relied on the assumption that the natural frequency ##\omega_n## is not equal to any of the frequencies present in the Fourier series of the input signal.

Thus, ##\omega_n\neq \omega, 2\omega, 3\omega, \ldots##.

If ##\omega_n## equals any of these frequencies then resonance occurs: the response is not periodic and the amplitude blows up in time.

If ##\omega_n## does not equal any of these frequencies then, as we see in (4), we have a periodic response.

At this point, I saw the following question:

Are there frequencies at which there is more than one periodic solution?

Here is the answer as it appears in these notes (answer to problem 5).


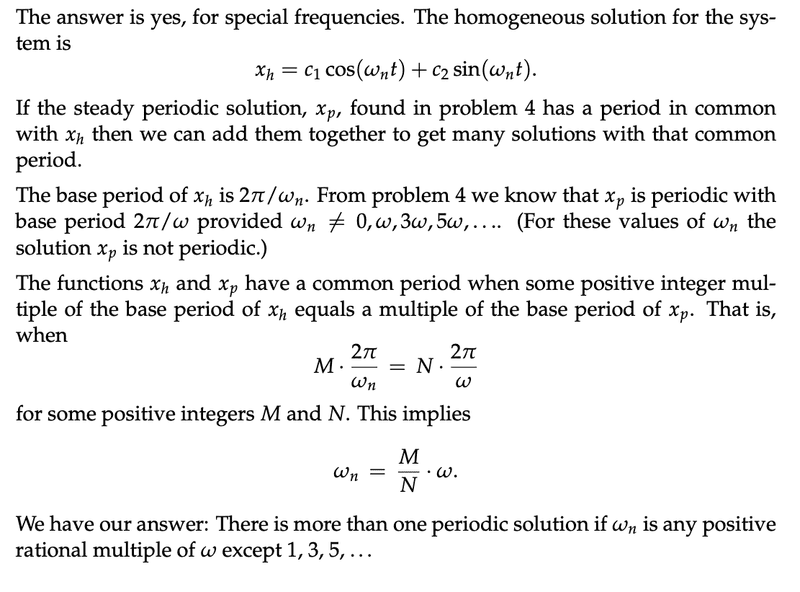
I didn't quite understand this.

Are we trying to specify an ##\omega_n## such that there are multiple different periodic ##x_p## that solve the differential equation?

Or are we after multiple different general solutions (ie, homogeneous solution plus inhomogeneous solution).

I don't think it is the latter option, since the homogeneous solution has constants so we already have infinite general solutions.

What exactly are we adding together "to get many solutions with that common period"?

zenterix said:

Are we trying to specify an ##\omega_n## such that there are multiple different periodic ##x_p## that solve the differential equation?

Yes.

For generic [itex]\omega_n[/itex] the general complementary function and the forcing function will not have a common period, so the motion is not periodic; the only periodic solution in that case is that obtained by taking the complementary function to be zero. Only when the general complementary function and the forcing function have a common period and the particular solution is not resonant are there multiple periodic solutions - every complementary function then gives rise to a different periodic solution.

@pasmith

Just to be clear we have

1) General complementary solution (to the homogeneous equation).
2) Particular solution (to the inhomogeneous equation).
3) General solution (to the inhomogeneous equation), sum of 1) and some solution from 2).

So you confirm that the multiple cases of periodic solutions for a specific ##\omega_n## occurs in case 3).

One reason I was a bit confused is because I thought that the entire analysis that I (and the books/notes I am reading) was doing was in the long-run, ie in the steady state, when the complementary solution is approximately zero.

Can I say, then, that in the long-run there are never multiple periodic solutions?

The complementary solution of [itex]\ddot x + \omega_n^2 x[/itex] does not decay. It has constant amplitude.

To get complementary solutions with decaying amplitude you must look at [tex]\ddot x + k\dot x + \omega_n^2 x = f(t)[/tex] for [itex]k > 0[/itex].

pasmith said:

The complementary solution of [itex]\ddot x + \omega_n^2 x[/itex] does not decay. It has constant amplitude.

That's true, what the heck am I saying.

Ok, I think I understand now.

Here is a summary of everything.

This question is from a problem set (and here are the solutions).

The questions basically ask us to drive a harmonic oscillator (natural frequency ##\omega_n##) with a triangle wave with angular frequency ##\omega## and find a periodic solution.

Periodic particular solution, that is.

If the forcing function angular frequency is fixed at ##\omega## and we can vary ##\omega_n## then we observe pure resonance when ##\omega_n## is made to be any of the frequencies present in the Fourier series of the forcing function.

If ##\omega_n## is not one of these special frequencies then "we get periodic solutions".

So, in fact, the problem set is speaking about particular solutions not the general solution (because for any one of these particular solutions, the general solution will only be periodic under special conditions described below).

Then, in the final problem (which is the question in the OP here), we are asked about ##\omega_n##'s that have multiple periodic solutions.

At this point, since we have been talking only about particular solutions, I think that we are talking about multiple periodic particular solutions (as confirmed by @pasmith)

Now, of course, if we add any homogeneous solution to a particular solution we get another particular solution.

However, if ##\omega## is a rational number multiple of ##\omega_n## (the frequency present in the homogeneous solution) then when we add the solutions not only will we have another particular solution, but it will be periodic.

Here is an example.

$$\ddot{x}+\omega_0^2x=g(t)$$

where

$$g(t)=\begin{cases} t\ \ \ \ \ -\frac{\pi}{2}\leq t\leq \frac{\pi}{2} \\ \pi-t\ \ \ \ \ \frac{\pi}{2}\leq t\leq \frac{3\pi}{2}\end{cases}$$

Using Fourier series we find a particular solution

$$x_p(t)=\frac{4}{\pi}\sum\limits_{k=0}^\infty \frac{(-1)^k}{(2k+1)^2}\frac{\sin{(2k+1)t}}{\omega_0^2-(2k+1)^2}$$

as long as ##\omega_0\neq 2k+1## for ##k\in\mathbb{N}_0##.

This particular solution is periodic. The smallest period is ##2\pi##.

The angular frequencies present in the response are ##\omega_r=1,3,5,7,\ldots##.

If ##\omega_0## equals any of these then we have pure resonance.

The homogeneous solution to the equation is

$$x_h(t)=c_1\cos{\omega_0 t}+c_2\sin{\omega_0 t}$$

We can add ##x_h(t)## to ##x_p(t)## to obtain another particular solution. This solution will only be periodic if ##\omega_0=r\omega_r## for some ##\omega_r=1,3,5,\ldots## and ##r\in\mathbb{Q}##.

For example, if ##\omega_0=\frac{4}{5}## then the natural period is ##2.5\pi##.

The particular solution

$$c_1 \cos{\left ( \frac{4t}{5} \right )}+c_2\sin{\left (\frac{4t}{5}\right )} +\frac{4}{\pi}\sum\limits_{k=0}^\infty \frac{(-1)^k}{(2k+1)^2}\frac{\sin{(2k+1)t}}{\omega_0^2-(2k+1)^2}$$

has smallest period of ##10\pi##.

It is periodic.

Note that for this given ##\omega_0## we have infinite possible periodic particular solutions.

If we were to choose ##\omega_0=\sqrt{2}## then there is no common period between ##x_h(t)=c_1\cos{\sqrt{2}t}+c_2\sin{\sqrt{2}t}## and ##x_p(t)## so ##x_h(t)+x_p(t)## in this case is not periodic.

For this choice of ##\omega_0## the only particular solution that is periodic is ##x_p(t)##.