- #1
Nova_Chr0n0
- 16
- 3
- Homework Statement
- Determine the reactions at the smooth contact points A, B, and C on the bar.
- Relevant Equations
- N/A
The figure is shown below:
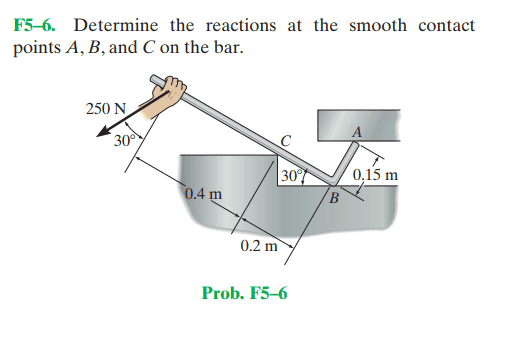
Here is my FBD for the figure with assign +x and +y directions
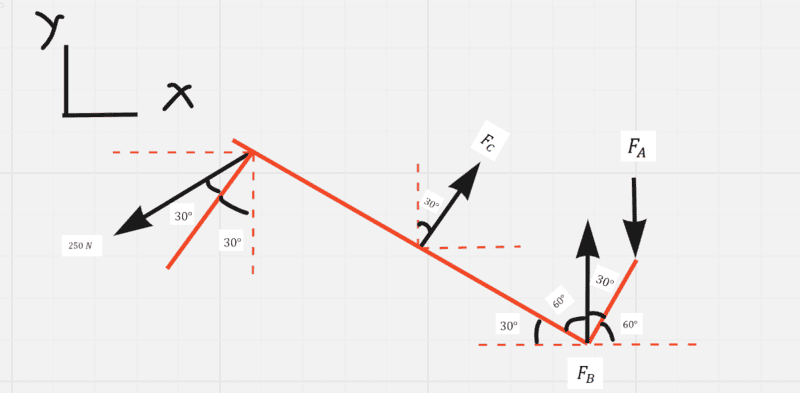
I started off by summing up the forces in the x-direction:
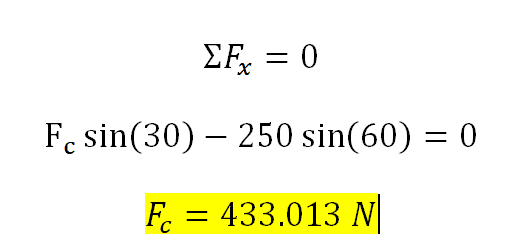
Next is the summing up of the forces in the y-direction:
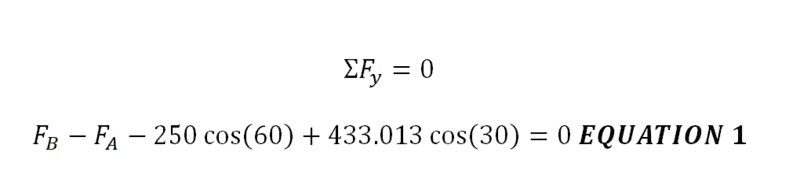
After this, I solved for the moment at point A: assuming that counter-clockwise is +
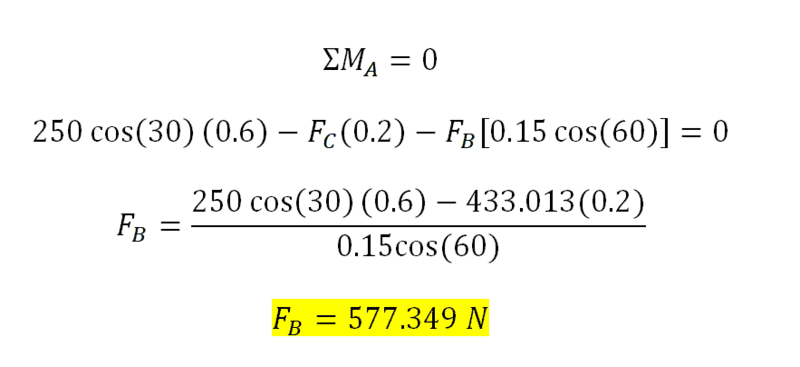
Now from equation 1, if I input the value of F_B, i would get F_A = 827.349 N.
Here is where my question starts. When I tried to search for the problem in the internet to double check the answers that I've got, I only got the Force C right. Mainly because most of them choose B as their moment point. Solution below:
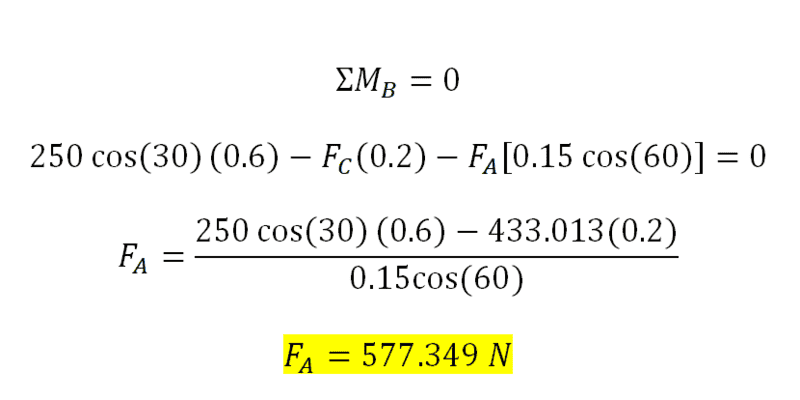
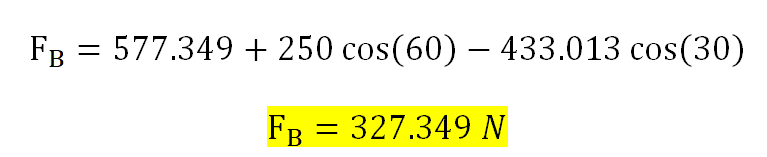
With this, I got two different values for Fa and Fb when choosing the moment point. I tried to find solution in the internet where they choose A as their moment point but I didn't finding anything. I tried to check my solution multiple times but I cannot find my mistake here. Is there something wrong with the values in my solution? Or is it wrong that I decided to choose A as my point of moment?
Here is my FBD for the figure with assign +x and +y directions
I started off by summing up the forces in the x-direction:
Next is the summing up of the forces in the y-direction:
After this, I solved for the moment at point A: assuming that counter-clockwise is +
Now from equation 1, if I input the value of F_B, i would get F_A = 827.349 N.
Here is where my question starts. When I tried to search for the problem in the internet to double check the answers that I've got, I only got the Force C right. Mainly because most of them choose B as their moment point. Solution below:
With this, I got two different values for Fa and Fb when choosing the moment point. I tried to find solution in the internet where they choose A as their moment point but I didn't finding anything. I tried to check my solution multiple times but I cannot find my mistake here. Is there something wrong with the values in my solution? Or is it wrong that I decided to choose A as my point of moment?