Signals and Systems: Determine the convolution of x(t) and h(t)

So, the convolution of two signals is described as follows:
$$ \int_{-\infty}^{\infty} x(\tau)h(t-\tau) \, d\tau $$
The figure shows the given signals. Now, as described in the convolution integral, I transformed ##h(t)## to ##h(-\tau)## by flipping the signal horizontally. So, now I have an impulse signal when ##\tau = -2##.

Now, the ##t## represents a horizontal shift in signal ##h(t-\tau)##. After which both signals are multiplied and then integrated. When, ##t<1##, then there is no overlap between ##h(\tau)## and ##x(\tau)##. The same is true when ##t>5##.
Now, I need to determine what the limits of the integration would be when there is an overlap between the two signals. If ##h(\tau)## were a rectangular signal, then it would be straightforward.


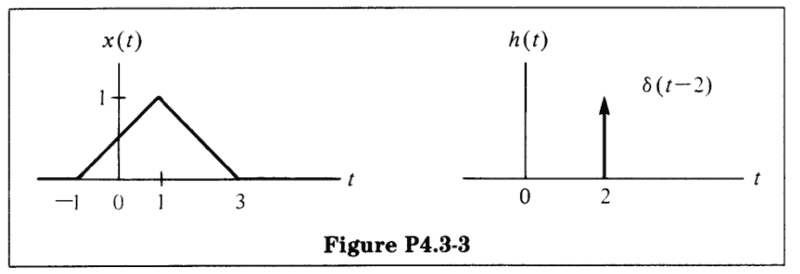
The impulse signal means the only overlap between the two signals exist at the exact point to where the impulse is shifted. Because of this, I don't know how to find the limits to evaluate the convolution integration. From what I have read online, I see that the output signal ##y(t)## is probably a horizontal shifted signal of ##x(t)## by 2 units to the right.

I can't seem to get the answer because I don't know what the limits of the integration are supposed to be. I thought the upper limit and the lower limit would be equal, but then the integration would evaluate to 0.

The limits are always from ##-\infty## to ##\infty##. It is not not very important for this example because ##h(t)## is only nonzero at one point. You do not need to consider the limits to evaluate the integral. You should look at where that point is involved in the convolution integral. That convolution is a function of ##t##.

DaveE said:

https://www.robots.ox.ac.uk/~dwm/Courses/2TF_2011/2TF-N3.pdf

That was very helpful, thank you!

FactChecker said:

The limits are always from ##-\infty## to ##\infty##. It is not not very important for this example because ##h(t)## is only nonzero at one point. You do not need to consider the limits to evaluate the integral. You should look at where that point is involved in the convolution integral. That convolution is a function of ##t##.

Makes sense now.
Thanks!