Higher Prequantum Geometry V: The Local Observables – Lie Theoretically
Show Complete Series
Part 1: Higher Prequantum Geometry I: The Need for Prequantum Geometry
Part 2: Higher Prequantum Geometry II: The Principle of Extremal Action – Comonadically
Part 3: Higher Prequantum Geometry III: The Global Action Functional — Cohomologically
Part 4: Higher Prequantum Geometry IV: The Covariant Phase Space – Transgressively
Part 5: Higher Prequantum Geometry V: The Local Observables – Lie Theoretically
Part 6: Examples of Prequantum Field Theories I: Gauge Fields
Part 7: Examples of Prequantum Field Theories II: Higher Gauge Fields
Part 8: Examples of Prequantum Field Theories III: Chern-Simons-type Theories
Part 9: Examples of Prequantum Field Theories IV: Wess-Zumino-Witten-type Theories
Part 10: Introduction to Perturbative Quantum Field Theory
This article discusses how the previous considerations naturally follow the concepts of local observables of local field theories and of the Poisson bracket on them; as well as that of conserved currents and the variational Noether theorem relating them to symmetries. At the same time, all these concepts are promoted to prequantum local field theory.
As throughout, all this is indebted to discussion with Igor Khavkine.
In the last two articles (the ones on the global action functional and on the covariant phase space) we have arrived at a perspective of prequantum local field theory where the input datum is a partial differential equation of motion ##\mathcal{E}## on sections of a bundle ##E## over spacetime/worldvolume ##\Sigma## and equipped with a prequantization exhibited by a factorization of the Euler-Lagrange form ##E \stackrel{\mathrm{EL}}{\longrightarrow} \mathbf{\Omega}^{p+1,1}_{S}## and of the presymplectic current form ##\mathcal{E} \stackrel{\Omega}{\longrightarrow} \mathbf{\Omega}^{p,2}## through higher Cech-Deligne cocycles for an Euler-Lagrange ##p##-gerbe ##\mathbf{L}## and for a Lepage ##p##-gerbe ##\mathbf{\Theta}##:
This local data then transgresses to spaces of field configurations over codimension-k submanifolds of ##\Sigma##. Transgressing to codimension-0 yields the globally defined exponentiated action functional
and transgressing to (the normal formal neighbourhood ##N^\infty_\Sigma \Sigma_p##of) a codimension-1 (Cauchy-)surface ##\Sigma_p \longrightarrow \Sigma## yields the covariant phase space as a prequantized pre-symplectic manifold
(Recall from the previous entry that throughout we are notationally abbreviating the sections of the shell ##\mathcal{E}## over the formal normal neighbourhood ##N^\infty_\Sigma \Sigma_p## of ##\Sigma_p## by suppressing the symbol for formal normals and just writing ##\Sigma## ##[\Sigma_p,\mathcal{E}]_\Sigma := [N^\infty_\Sigma \Sigma_p, \mathcal{E}]##.)
Given any space equipped with a map into some moduli space like this, an automorphism of this structure is a diffeomorphism of the space together with a homotopy which exhibits the preservation of the given map into the moduli space.
We consider now the automorphisms of the prequantized covariant phase space and of the Euler-Lagrange ##p##-gerbe that it arises from via transgression and find that these recover and make globally well-defined the traditional concepts of symmetries and conserved currents, related by the Noether theorem, and of observables equipped with their canonical Poisson bracket.
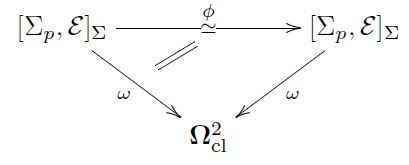
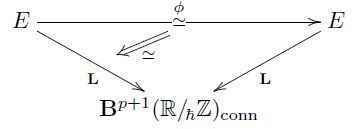
The correct automorphisms of presymplectic smooth spaces ##([\Sigma_p,\mathcal{E}]_\Sigma,\omega) \longrightarrow ([\Sigma_p,\mathcal{E}]_\Sigma,\omega)## are of course diffeomorphisms ##\phi : [\Sigma_p,\mathcal{E}]_\Sigma \longrightarrow [\Sigma_p,\mathcal{E}]_\Sigma## such that the presymplectic form is preserved, ##\phi^\ast \omega = \omega##. In the diagrammatics this means that ##\phi## fits into a triangle of the form
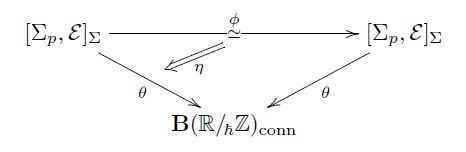
Viewed this way, there is an evident definition of an automorphism of a prequantization ##([\Sigma_p,\mathcal{E}]_\Sigma,\mathbf{\theta})## of ##([\Sigma_p,\mathcal{E}]_\Sigma,\omega)##. This must be a diagram of the following form
hence a diffeomorphism ##\phi## together with a homotopy ##\eta## that relates the modulating morphism of the translated prequantum bundle back to the original prequantum bundle. By the discussion in a previous article such homotopies are equivalently coboundaries between the Cech-Deligne cocycles that correspond to the maps that the homotopy goes between. Here this means that the homotopy in the above diagram is an isomorphism ##\eta : \phi^\ast \mathbf{\theta} \stackrel{\simeq}{\longrightarrow} \mathbf{\theta}## of circle bundles with connection. These pairs ##(\phi,\eta)## is what Souriau called the quantomorphisms. Via their canonical action on the space of sections of the prequantum bundle, these become the quantum operators.
To see what this is in local data, consider the special case that ##\theta## is a globally defined 1-form and suppose that ##\phi = \exp(t v)## is the flow of a vector field ##v##
Then the homotopy filling the previous diagram is given by a smooth function ##\exp(i t \alpha )## such that
$$
\exp(t v)^\ast \theta – \theta = t d \alpha
\,.
$$
Infinitesimally, for ##t \to 0##, this becomes
$$
\mathcal{L}_v \theta = d \alpha
\,.
$$
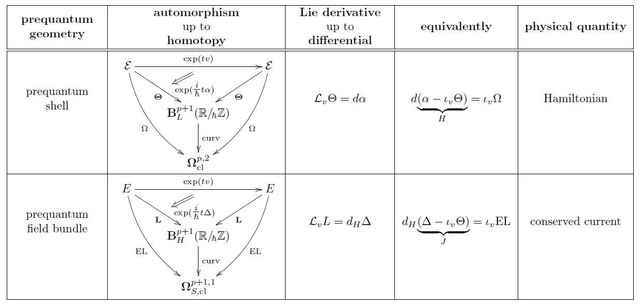
Using Cartan’s formula for the Lie derivative on the left, and the fact that ##d \theta = \omega##, by prequantization, this is equivalent to
$$
d \underset{H}{\underbrace{( \alpha – \iota_v \theta )}} = \iota_v \omega
$$
This is the classical formula [Arnold 89] which says that
$$
H := \alpha – \iota_v \theta
$$
is a Hamiltonian for the vector field ##v##.
There is an evident smooth group structure on the homotopies as above, and one check that the induced Lie bracket on Hamiltonians ##H## with Hamiltonian vector fields ##v## is the following
$$
[(v_1,H_1), (v_2,H_2)] = ( [v_1,v_2], \iota_{v_2}\iota_{v_1}\omega )
\,.
$$
Traditionally this is considered only in the special case that ##\omega## is symplectic, hence equivalently, in the case that the above equation for ##d H## uniquely associates a Hamiltonian vector field ##v## with any Hamiltonian ##H##. In that case, we may identify a pair ##(v,H)## with just ##H## and then the above Lie bracket becomes the Poisson bracket on smooth functions induced by ##\omega##. Hence the Poisson bracket Lie algebra is secretly the infinitesimal symmetries of the prequantum line bundle ##\mathbf{\theta}##.
This is noteworthy. For instance in the example of the phase space ##(T^\ast \mathbb{R} = \mathbb{R}^2, \omega = d p \wedge dq)##
and writing ##q,p : \mathbb{R}^2 \to \mathbb{R}## for the two canonical coordinates (##p## is called the “canonical momentum”), then the Poisson bracket, as above, between these two is
$$[q,p] = i \hbar \;\;\; \in \;\; i \hbar \mathbb{R} \hookrightarrow \mathfrak{Pois}(\mathbb{R}^2,d p \wedge d q)\,.$$
This equation is often regarded as the hallmark of quantum theory. In fact, it is a prequantum phenomenon. Notice how the identification of the central term with ##i \hbar## follows here from the first prequantization step back in an earlier entry.
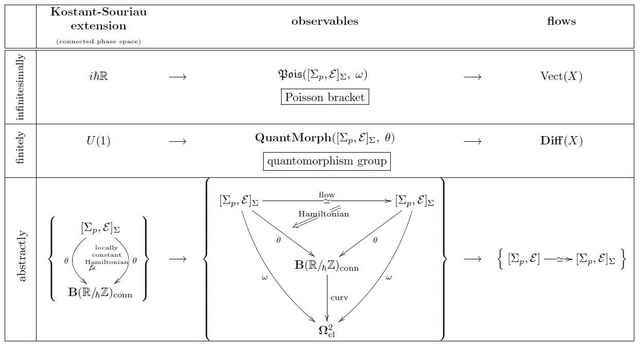
From the above equation for ##d H## it is clear that the Poisson bracket is a Lie extension of the Lie algebra of (Hamiltonian) vector fields by the locally constant Hamiltonians, hence by constant functions in the case that ##X## is connected. The non-trivial Lie integration of this statement is the Kostant-Souriau extension, which says that the quantomorphism group of connected phase space is a ##U(1)##-an extension of the diffeological group of Hamiltonian symplectomorphisms.
Hence in summary the situation for observables on the covariant phase space in codimension 1 is as follows:
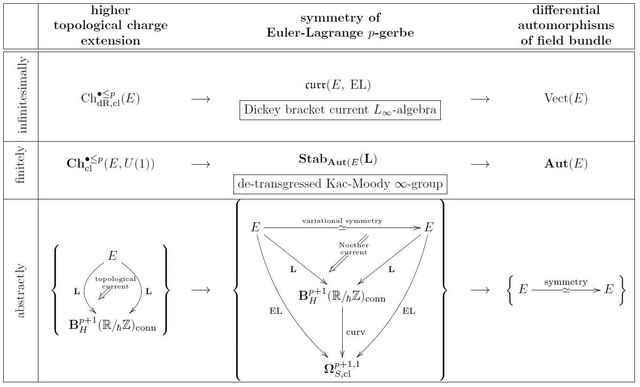
The above diagrammatic incarnation of the quantomorphism group and of the Poisson bracket has the advantage that it makes sense in more generality. The same kind of diagrams, but with the prequantum bundle ##\theta## replaced by the Lepage ##p##-gerbe that it arises from via transgression, gives a “de-transgression” of the Poisson bracket on observables to a higher bracket on “local observables”, namely on currents that give observables after integration over Cauchy surfaces, and more generally on higher currents that give observables after integration over higher codimension subspaces of spacetime/worldvolume.
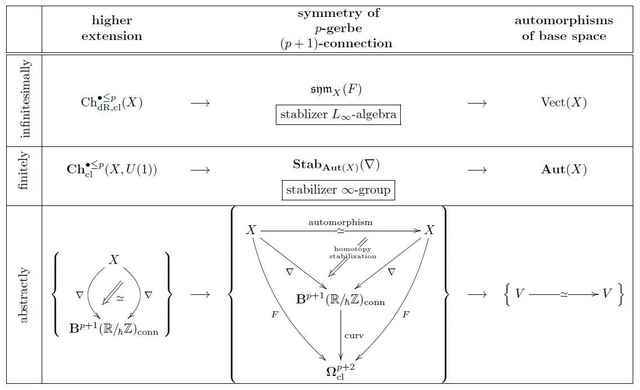
Moreover, generally the symmetries of a ##p##-gerbe connection ##\nabla : X \to \mathbf{B}^{p+1} (\mathbb{R}/_{\!\hbar}\mathbb{Z})_{\mathrm{conn}}## form an extension of the symmetry group of the underlying space by the higher group of flat ##(p-1)##-gerbe connections [Fiorenza-Rogers-Schreiber 13a]:
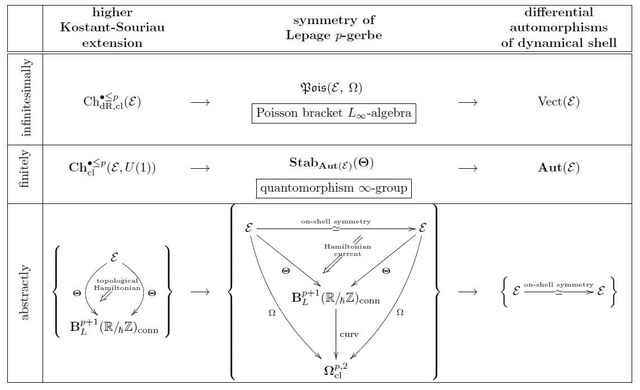
Specifying this general phenomenon to the Lepage ##p##-gerbes, it gives a Poisson bracket ##L_\infty##-algebra on higher currents (local observables) [Fiorenza-Rogers-Schreiber 13b] and its higher Lie integration to a higher quantomorphism group constituting a higher Kostant-Souriau extension of the differential automorphisms of the field bundle. This is determined by the (pre-)symplectic current ##p+2##-form ##\Omega## in analogy to how the ordinary Poisson bracket is determined by the (pre-)symplectic 2-form ##\omega##, hence this is a Poisson ##L_\infty##-bracket for what has been called “multisymplectic geometry” (see [Rogers 10]):
So far this concerned the covariant phase space with its prequantization via the Lepage ##p##-gerbe. In the same way, there are the higher symmetries of the field space with its prequantization via the Euler-Lagrange ##p##-gerbes ##\mathbf{L}##:
To see what these are in components, consider again the special case that ##\mathbf{L}## is given by a globally defined horizontal form, and consider a one-parameter flow of such symmetries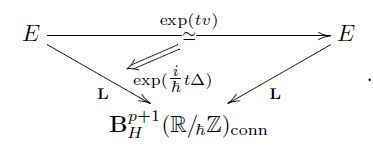 In Cech-Deligne cochain components this diagram equivalently exhibits the equation
In Cech-Deligne cochain components this diagram equivalently exhibits the equation
$$
\exp(t v)^\ast L – L = t \, d_H \Delta
$$
on differential forms on the jet bundle of ##E##, where ##v## is a vertical vector field. Infinitesimally for ##t\to 0## this becomes
$$
\mathcal{L}_v L = d_H \Delta
\,.
$$
Since ##L## is horizontal while ##v## is vertical, the left hand reduces, by the central equation of a previous article, to
$$
\iota_v d L = \iota_v( \mathrm{EL} – d_H\Theta )
\,,
$$
Therefore the infinitesimal symmetry of ##\mathbf{L}## is equivalent to
$$
d_H \underbrace{( \Delta – \iota_v \Theta )}_{J} = \iota_v \mathrm{EL}
\,.
$$
This says that associated to the symmetry ##v## is a current
$$
J := \Delta – \iota_v \Theta
$$
which is conserved (horizontally closed) on the shell (on the vanishing locus ##\mathcal{E}## of the Euler-Lagrange form ##\mathrm{EL}##).
This is precisely the statement of Noether’s theorem (the first variational theorem of Noether, to be precise).
Indeed, in its modern incarnation [Vinogradov 86, Khavkine 16], Noether’s theorem is understood as stating a Lie algebra extension of the Lie algebra of symmetries by topological currents to the Lie-Dickey bracket on equivalence classes of conserved currents (see [Barnich-Henneaux 96, section 3]).
Hence the ##\infty##-group extension of symmetries of the Euler-Lagrange ##p##-gerbe promotes Noether’s theorem to the statement that higher Noether currents form an ##L_\infty##-algebra extension of the infinitesimal symmetries by topological currents:
In summary, physical local observables arise from symmetries of higher prequantum geometry as follows:
That’s a fair bit of classical mechanics and of classical local field theory which we have now re-derived and pre-quantized. It’s time for some examples. The next two articles will be about applying all this to field theories of higher Wess-Zumino-Witten type and to field theories of higher Chern-Simons type.
I am a researcher in the department Algebra, Geometry and Mathematical Physics of the Institute of Mathematics at the Czech Academy of the Sciences (CAS) in Prague.
Presently I am on leave at the Max Planck Institute for Mathematics in Bonn.



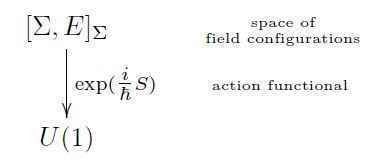

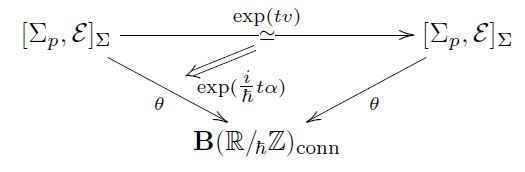




Speaking of geometric concept
I add here a link Lighting engaged in
ElectroGravity Via Geometric TimeField
[MEDIA=youtube]coMaozR9vY8[/MEDIA]