Is Mechanical Energy Conservation Free of Ambiguity?

Introduction

“Close to any question that is in the textbook, there is another question that has never been answered that is interesting.”
[Stephen Wolfram, remarks to The University of Vermont physics students, September 30, 2005]

Mechanical energy conservation is the assertion that the sum of kinetic and potential energies of a system (the mechanical energy) does not change as a mass moves from point A to point B. Conventionally we may write
$$K_A+U_A=K_B+U_B~~~~~(\rm{I.1a})$$which can be rewritten in a form that does not require specifying the “zero of energy”, $$\Delta K+\Delta U=0~~~~~(\rm{I.1b})$$Equation (I1.b) has found extensive use as a problem-solving technique in cases where the SUVAT equations do not apply, e.g. a roller coaster, in which one needs to relate position and speed at point A with position and speed at point B.  A familiar textbook  derivation of this relies on the work-energy theorem (W-E theorem) which itself is derived from Newton’s second law:

$$\vec F_{net}=m\vec a$$

$$\vec F_{net}\cdot \vec v=m\vec a\cdot \vec v=m\vec v\cdot \frac{d\vec v}{dt}=\frac{1}{2}m\frac{d}{dt}(\vec v\cdot \vec v)=\frac{d}{dt}\left(\frac{1}{2}mv^2 \right)=\frac{dK}{dt}.$$

It follows that $$dK=\vec F_{net}\cdot \vec v dt=\vec F_{net}\cdot d\vec s.$$ Integrating, $$\int_A^B dK= \Delta K =\int_A^B \vec F_{net}\cdot d\vec s~~~~~(\rm{I.2})$$and with $$W_{net}=\int_A^B \vec F_{net}\cdot d\vec s,$$we obtain the work-energy theorem, $$\Delta K=W_{net}~~~~~(\rm{I.3})$$Continuing on with standard practice, we separate the net work into work done by conservative forces and work done by non-conservative forces in which case,$$\Delta K=W_{cons.}+W_{non\text{-}cons}. $$ Beyond this, we use the definition of potential energy change as the negative of the work done on the system by all the conservative forces to obtain,$$\Delta K+\Delta U=W_{non\text{-}cons}~~~~~(\rm{I.4})$$Clearly, when the work done by non-conservative forces is zero, the W-E theorem (I.4) reduces to the statement of mechanical energy conservation, equation (I.1b).  Therefore, a logical criterion for MEC is “Mechanical energy is conserved when the net work done by all non-conservative forces on a system or its components is zero.”  Although this statement makes sense in view of equation (I.4), it is problematic. As we shall see, although dissipative forces are non-conservative, they do not do mechanical work and therefore the energy transformations they effect cannot be placed under the ##W_{non\text{-}cons}## umbrella.

This is the first article in a three-part series focused on the use of MEC in introductory physics pedagogy, specifically problem-solving related to mechanical energy conservation.  It is an attempt to make the case that MEC and the W-E theorem leave a lot to be desired in terms of clarity.  Part II is an examination of how the law of energy conservation can be extended to apply to cases involving dissipative forces where MEC is inapplicable as a problem-solving method.  In part III the merits of modifying, replacing, or leaving MEC alone are briefly discussed.

When is mechanical energy conserved?

Here is a multiple-choice question for the reader.  The figure below shows the free body diagram of a block being acted upon by non-zero resultant force ##\vec F_{net}##.  The block’s velocity is in the direction of the force.  Question: Is mechanical energy conserved?

$$\text{(a) Yes.          (b) No.          (c) Maybe.}$$

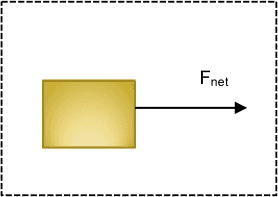
I suspect most readers will select (c) by considering, within the parameters of the question, one case where (a) is unequivocally the answer and a counterexample where (b) is unequivocally the answer.  For example, imagine that the block is sliding down on an inclined plane near the Earth’s surface.  Relative to the direction of ##\vec F_{net}##, gravity acts at an acute angle while the normal force is perpendicular.  If there is no friction, mechanical energy is conserved; if there is friction, mechanical energy is not conserved.  Therefore, (c) is the correct answer.

However, the correct answer is (b).   The justification has nothing to do with the nature of the various forces that are added to give the resultant ##\vec F_{net}##.  It can be summarized by “because we have a single-component system that is not isolated.”  This point will be clarified in the next section when we bring forth the importance of the system’s boundary (dashed rectangle)  and argue that the nature of the forces acting on the system alone is insufficient to determine whether mechanical energy is conserved.

Most introductory physics textbooks include the circumstances under which MEC may or may not be used.  A survey of textbooks on my shelves produced the following, with some variations in language

ME is conserved when

1. Only conservative forces act on the system or its internal components.
2. No non-conservative forces act on the system or its internal components.
3. Only conservative forces do work on the system or its internal components.

Criteria 1 and 2 are perfectly understandable when one considers equation (I.2).  If ##\vec F_{net}## is the sum of only conservative forces, then these forces can be derived from potentials in which case the integral on the right-hand side of the equation can be moved to the left side with a sign change and appear as a change in total potential energy.  Criterion 3 improves 1 and 2 by taking into account non-conservative forces that do no work, e.g the tension in the case of a pendulum or the normal force in the case of an ideal roller coaster.

However, these criteria have the problem that strict adherence to them necessitates dismissal of the application of MEC in cases where non-conservative forces do work on the system or its internal components.   For example, if we consider the “system” to be one of the masses in an ideal Atwood machine, we must conclude that MEC is inapplicable, yet it is widely applied in textbook examples and we all agree that MEC is an appropriate problem-solving technique in the Atwood machine case.  However, the message sent to the novice, who may be trying to understand how the criteria are applied, is that either the criteria do not really matter or that tension is a conservative force.

Textbooks and websites advocating the use of MEC while contradicting their own criteria cannot be effective in conveying to novices an understanding of when mechanical energy is conserved.  Of course we, the experts, “know” when mechanical energy is conserved and confidently encourage novices to use MEC rarely bothering to establish whether the criteria are fulfilled. But what is it that we know?  We know that MEC is a special case of the law of conservation of energy.  We also know that mechanical energy is conserved when dissipative forces are absent from energy transformations within a closed system.  We may not expressly state so, but at some level, we understand that mechanical energy is conserved when the mass moving from point A to point B does so reversibly in the thermodynamic sense.

A cursory web search on “When is mechanical energy conserved?” yielded a multitude of hits, the first six of which are shown in the Appendix.  Of these 1 and 3 enunciate that mechanical energy is not conserved when dissipative forces come into play.  Statement 3 gives examples of dissipative forces and says that mechanical energy is conserved “as long as we ignore” them.  This should be taken to mean “as long as their effects are negligible relative to non-dissipative energy transformations”.    However the use of  “outside” instead of “dissipative” to characterize the forces is unfortunate.  The remaining four focus on the distinction between conservative and non-conservative and, in my opinion, miss the mark.  The reader is encouraged to evaluate the extent to which these sites and others provide a uniform take-home message to a novice trying to sort out when mechanical energy is conserved.

The importance of system boundaries

We note that ##W## in the work-energy theorem ought to be thermodynamic work, the same ##W## that appears in the first law of thermodynamics.  Just because no heat is added to a mass in free fall (##Q = 0##) it doesn’t mean that the work done by gravity on the mass is some different kind of work or that the mass does not obey the law of energy conservation exemplified by the first law.  Percy Bridgman has sharpened our understanding in this regard.

Turn now to an examination of the ##W## of the First Law.  This ##W## means the total mechanical work received by the region inside the boundary from the region outside (or delivered from the region inside to the region outside).  As in the case of ##Q##, this work is done across the boundary, and the evaluation of ##W## demands the posting of sentries at all points of the boundary, and the summing of their contributions.  In the simple cases, usually considered in elementary discussions, the work received by the inside from the outside is of the simple sort typified by the motion of stretched cords or of simple piston rods.  Our sentry can adequately report this sort of thing in terms of finite forces acting at points and finite displacements. [P.W. Bridgman, The Nature of Thermodynamics, Harvard University Press, p. 30, (1941).]

To reduce ambiguity, one must have a clear definition of the system boundary because it separates external from internal forces.  For example, the statement “the mechanical energy of the roller coaster at point A is the same as the mechanical energy of the roller coaster at point B”, equation (I.1a), implies that the system is the roller coaster which “has” potential and kinetic energy at two different points along its path such that the sum of the two is invariant.  This is an inappropriate consideration.

If the system consists of a single component, the roller coaster, then the Earth increases or decreases this system’s kinetic energy by doing positive or negative external work on it.  A single-component system does not have potential energy, only kinetic (think of the degrees of freedom of an ideal monatomic gas then reduce the number of atoms to 1.)  Therefore, when external work is done on such a system, its kinetic energy changes and the system’s mechanical energy is not conserved.  The reader will note that none of the criteria listed here or in the Appendix require that the system have at least two components as a precondition for mechanical energy conservation.  Inattention to detail fosters a lack of clarity and spawns bewilderment.

Work-energy theorem shortcomings

In the words of Arnold Arons, “Because work, as a quantity of energy transferred in accordance with the First Law of Thermodynamics, cannot be calculated in general as an applied force times the displacement of the center of mass, the work-energy theorem is not a valid statement about energy transformations when work is done against a frictional force or actions on or by deformable bodies.” [A. B. Arons, Am. J. Phys., 67, 1063, (1999).]  It is best to use specific examples to illustrate what Arons is talking about.

I. Work done against friction

Consider the problem of a billiard ball of radius ##R## spinning in the air with initial angular speed ##\omega_0##. The ball falls straight down and lands on a pool table. At first the ball slips and rolls but eventually it starts rolling without slipping. (a) Find the linear speed of the ball when it starts rolling without slipping;(b) Find the distance the center of mass has traveled and the angle by which it has turned from impact until it rolls without slipping. Ignore rolling friction.

We ask the question, what the work-energy theorem has to say in this case? (The solution to the problem will be offered in part II.) The change in kinetic energy on the left hand side is $$\Delta K=\frac{1}{2}I_{cm}(\omega_f^2-\omega_0^2)+\frac{1}{2}mV_{cm}^2$$where ##I_{cm}## is the moment of inertia about the center of mass and ##V_{cm}=\omega_f R##. What about ##W_{net}## on the right-hand side of the W-E theorem? Friction is the only horizontal force acting on the ball while the vertical forces cancel. Therefore friction is the net force and is responsible for the gain in kinetic energy of the CM. It seems logical to conclude that ##W_{net}=\vec f_k \cdot \Delta \vec s_{cm}## because what else is there to say?. This is exactly the kind of “logic” that Arons warns against. The ##\vec f_k \cdot \Delta \vec s_{cm}## term is responsible for increasing the kinetic energy of the center of mass. However, within the confines of the W-E theorem, there is no term to account for the decrease of the spin kinetic energy of the ball. What else there is to say is that Bridgman’s sentries report that additional mechanical work ##\Delta W=\text{-}f_k R\Delta \theta## is converted by friction into heat on the periphery of the system boundary. Since ##\vec f_k##, in this case, is in the same direction as the velocity of the CM, one ought to write the right-hand side as shown below, $$\Delta K=f_k \Delta s_{cm}-f_k R\Delta \theta.$$Note that when the ball rolls with slipping, (##\Delta s_{cm}<R\Delta \theta~##) the kinetic energy decreases; when it rolls without slipping, (##\Delta s_{cm}=R\Delta \theta~##), the kinetic energy does not change.

(Note to the reader:  This section has been revised following a spirited discussion about it.)

II. Actions on or by deformable bodies

The figure shows a jumping gymnast (the system).  She starts from a crouching position (left) flexes her muscles and is at the point of just losing contact with the floor (right). What can the work-energy theorem say in this case? Clearly, the gymnast acquires kinetic energy (##\Delta K \neq 0##), but what net force is responsible for ##W_{net}##?

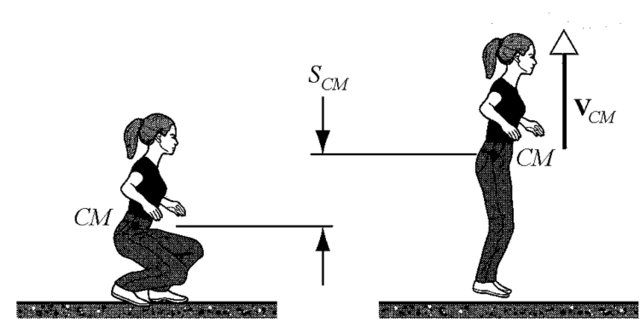
In this example, the acquisition of kinetic energy by a deformable body cannot be equated to work done by a net force as the work-energy theorem requires.

Remarks

To summarize, it looks like the derivation of MEC and the W-E theorem from Newton’s Law just don’t fit quite right.  The problem seems to stem from lumping together dissipative with non-conservative forces.   Everything is fine up to equation (I.2).  Given the definition of kinetic energy, the left side is an exact differential and is written as ##\Delta K## upon integration.  Difficulties arise when, on the right-hand side, line integrals are identified as work done on the system without giving special consideration to dissipative forces.  The derivation of the W-E theorem from Newton’s second law permits the “adulteration” of ##W_{net}## in equation (I.3)  with dissipative forces that convert mechanical work into heat but do no thermodynamic work.  Furthermore,  the role of non-conservative non-dissipative force, e.g. static friction and tension, that convert mechanical energy from one form into another without generating heat is not recognized as conserving mechanical energy.

In the next article in the series, Can We Do Better than Mechanical Energy Conservation? we will explore how Bridgman’s and Arons’s ideas can be incorporated into an alternate strategy for introductory physics problem-solving that relies on the law of energy conservation rather than mechanical energy conservation and the work-energy theorem.    All systems obey the law of energy conservation, sometimes formulated as the first law of thermodynamics.  It matters not whether such systems appear in a textbook’s  chapter 8, “Conservation of Energy”, or chapter 20, “The first law of Thermodynamics.” The law of energy conservation spans across the artificial boundaries of chapter headings.

In the third article of the series, Why Bother Teaching Mechanical Energy Conservation? we will see a less radical approach that addresses the flaws and ambiguity seen here.  It is a compromise between the two extremes that preserves mechanical energy conservation and modifies the work-energy theorem within the framework of the law of energy conservation.

Comment Thread

References

1. Law of Conservation of Mechanical Energy: The total amount of mechanical energy, in a closed system in the absence of dissipative forces (e.g. friction, air resistance), remains constant.
https://www.siyavula.com/read/science/grade-10/mechanical-energy/22-mechanical-energy-05

2. The principle of the conservation of mechanical energy states that the total mechanical energy in a system (i.e., the sum of the potential plus kinetic energies) remains constant as long as the only forces acting are conservative forces. We could use a circular definition and say that a conservative force as (sic) a force which doesn’t change the total mechanical energy, which is true but might shed much light on what it means.
http://physics.bu.edu/~duffy/py105/EnergyConservation.html

3. Mechanical energy is conserved so long as we ignore air resistance, friction, etc. When we don’t ignore outside forces, such as those just mentioned, mechanical energy is not conserved.
https://socratic.org/questions/is-mechanical-energy-always-conserved-why-or-why-not

4. The principle of conservation of mechanical energy states that in an isolated system that is only subject to conservative forces, the mechanical energy is constant.
https://en.wikipedia.org/wiki/Mechanical_energy

5. The principle (of conservation of mechanical energy) says that if the net work done by nonconservative forces is zero, the total mechanical energy of an object is conserved; that is, it doesn’t change.
https://www.dummies.com/education/science/physics/the-principle-of-conservation-of-mechanical-energy/

6. In special cases where energy is not lost to the environment due to nonconservative forces such as friction, the mechanical energy of a system of masses remains constant as the object or objects move in space. In other words, the energy of the system stays the same for all times in the life of the objects.
https://www.chipola.edu/instruct/science/Bodart/1121workenergy.htm