- #1
sol47739
- 38
- 3
- TL;DR Summary
- Can you by any type of rotations or transformations turn a left handed helix into a right handed or vice versa?
Can you by any type of rotations or transformations turn a left handed helix into a right handed or vice versa? If yes why? And if no, why not?
For example if you have a 2 d triangle like the one in the picture:
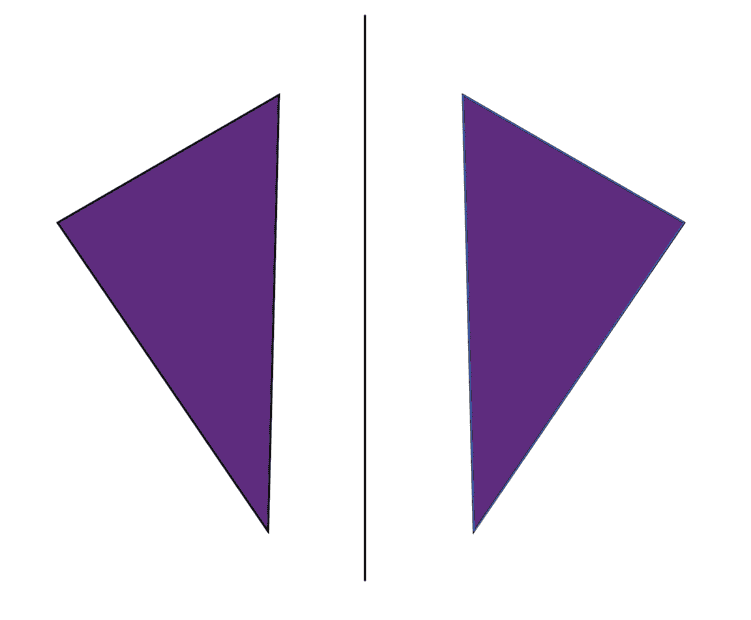
You can turn it in 3 dimensions 180 degrees in a sense mirroring it and you will get back to the original, but then you have to turn it in 3 dimension in a sense lift it up from the plane and turn it.
Can you do the same for a helix or not? If you were able to turn it any way you wanted in 3 dimensions as many times as you wanted could you get a left handed screw to become a right handed?
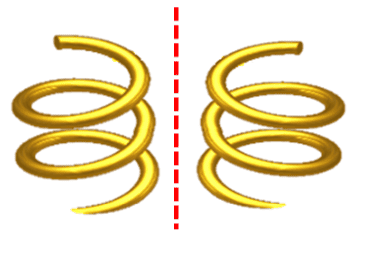
For example if you have a 2 d triangle like the one in the picture:
You can turn it in 3 dimensions 180 degrees in a sense mirroring it and you will get back to the original, but then you have to turn it in 3 dimension in a sense lift it up from the plane and turn it.
Can you do the same for a helix or not? If you were able to turn it any way you wanted in 3 dimensions as many times as you wanted could you get a left handed screw to become a right handed?