Exploring Proposition 6.1.2 from D&K's Multidimensional Real Analysis II (Integration)

• Feb 11, 2022

• #1

Math Amateur

Gold Member

MHB

3,990

48

I am reading Multidimensional Real Analysis II (Integration) by J.J. Duistermaat and J.A.C. Kolk ... and am focused on Chapter 6: Integration ...

I need some help with the proof of Proposition 6.1.2 ... and for this post I will focus on the first auxiliary result ... see (i) ... at the start of the proof ...Near the start of the proof of Proposition 6.1.2 D&K state that :

" ... ... Because b_j - a_j = (b_j - t_j) + (t_j - a_j), it follows straight away that :

\(\displaystyle \text{ vol}_n (B) = \text{ vol}_n (B') + \text{ vol}_n (B'') \)Readers of this post only need to read the very first part of the proof of Proposition 1 (see scanned text below) ... BUT ... I am providing a full text of the proof together with preliminary definitions so readers can get the context and meaning of the overall proof ... but, as I have said, it is not necessary for readers to read any more than the very first few lines of the proof.
Can someone please help me to rigorously prove that \(\displaystyle \text{ vol}_n (B) = \text{ vol}_n (B') + \text{ vol}_n (B'') \) ...Hope someone can help ...

Help will be much appreciated ...

PeterThe proof of Proposition 6.1.2 together with preliminary notes and definitions reads as follows:

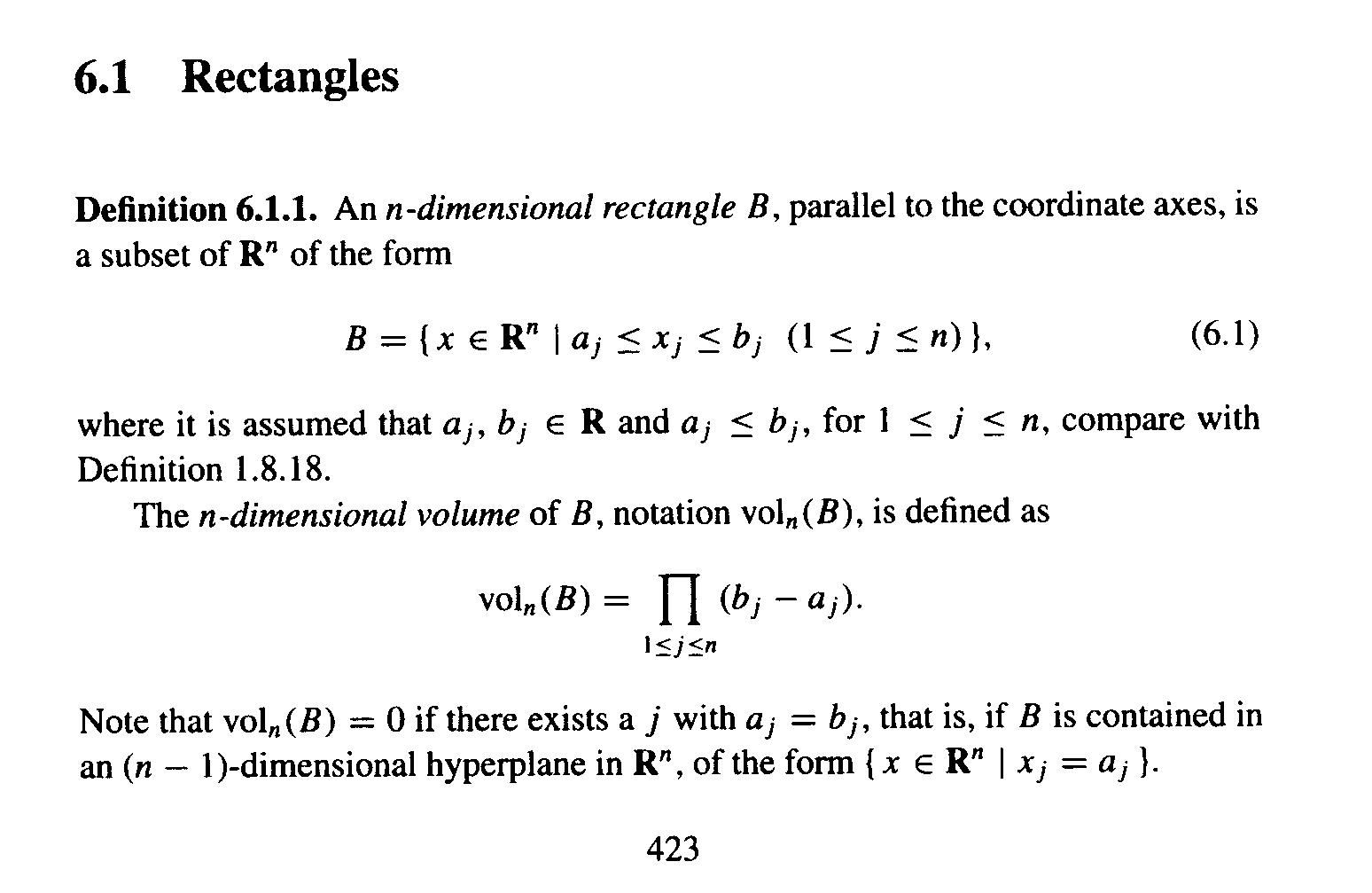
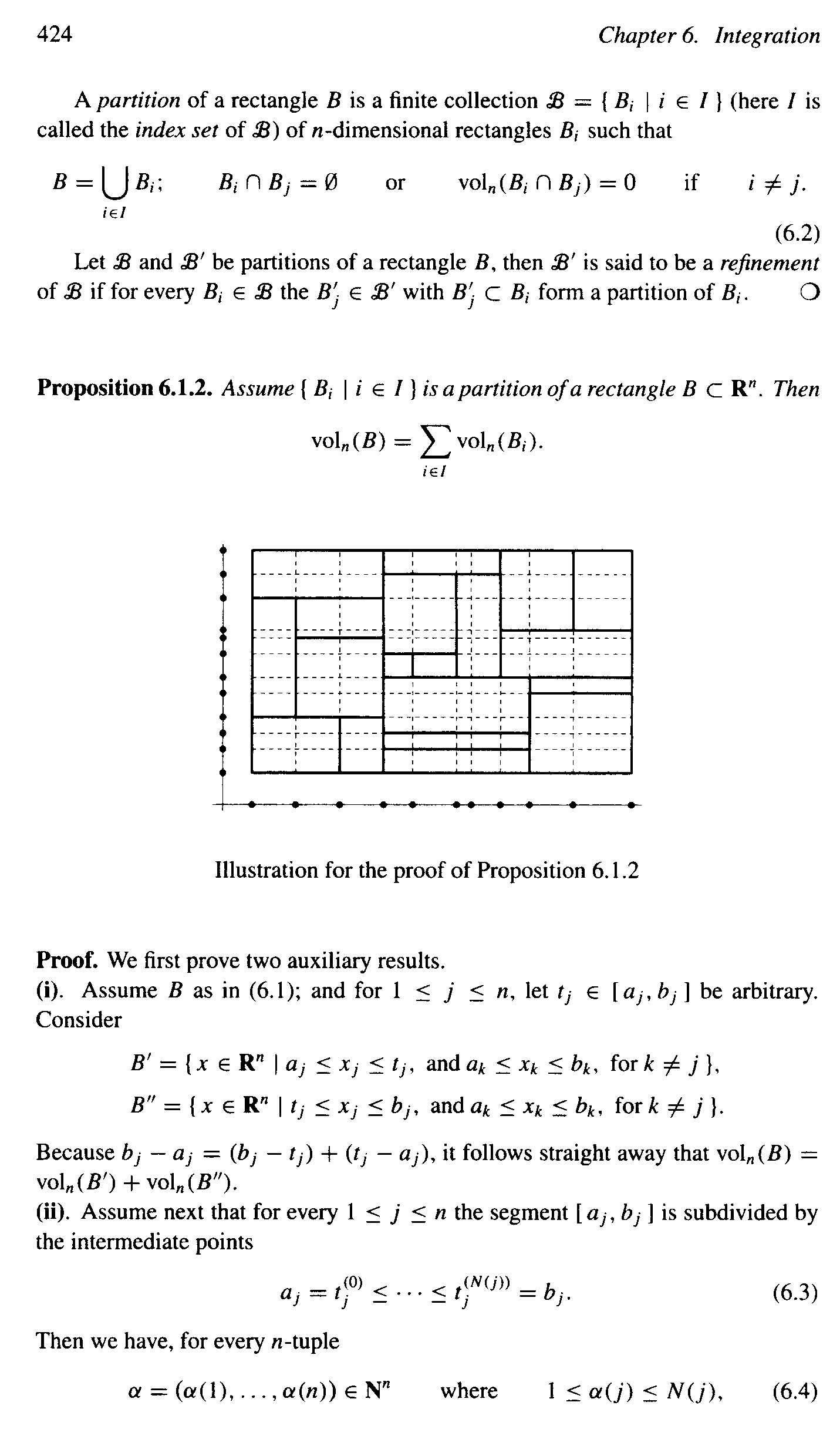
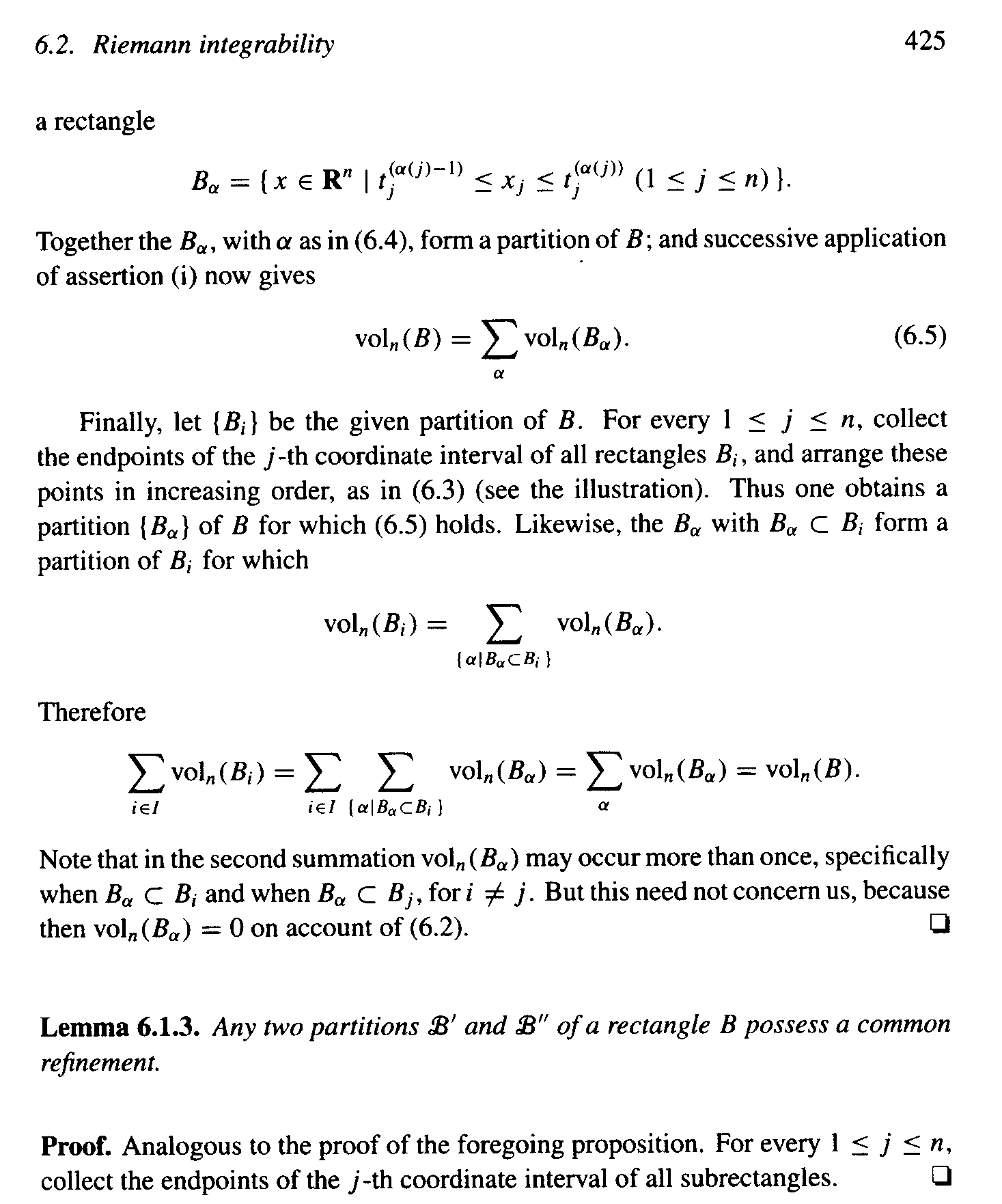Hope that helps,

Peter

 

Last edited: Feb 11, 2022

Physics news on Phys.org

• Research suggests how turbulence can be used to generate patterns
• High-quality microwave signals generated from tiny photonic chip
• Quantum talk with magnetic disks

• May 31, 2022

• #2

Euge

Gold Member

MHB

POTW Director

2,048

195

Hi Peter,

By definition of volume, $$\text{vol}_n(B') = (t_j - a_j)\prod_{k \neq j} (b_k - a_k)\quad \text{and}\quad \text{vol}_n(B'') = (b_j - a_j) \prod_{k \neq j} (b_k - a_k)$$
Hence the sum $$\text{vol}_n(B') + \text{vol}_n(B) = [(t_j - a_j) + (b_j - a_j)] \prod_{k \neq j} (b_k - a_k) = (b_j - a_j)\prod_{k \neq j} (b_k - a_k) = \prod_k (b_k - a_k) = \text{vol}_n(B)$$ as desired.

 

• Feb 11, 2023

• #3

soloenergy

64

0

Preliminary notes and definitions:

Let B = [a_1, b_1] \times [a_2, b_2] \times ... \times [a_n, b_n] be a closed n-dimensional rectangle in \mathbb{R}^n. We define the volume of B as:

\text{vol}_n (B) = (b_1 - a_1)(b_2 - a_2) ... (b_n - a_n)

Now, let t_j \in [a_j, b_j] for j = 1, 2, ..., n. We define the rectangles B' = [a_1, t_1] \times [a_2, t_2] \times ... \times [a_n, t_n] and B'' = [t_1, b_1] \times [t_2, b_2] \times ... \times [t_n, b_n].

Proposition 6.1.2: Let B, B', B'' be as defined above. Then, \text{ vol}_n (B) = \text{ vol}_n (B') + \text{ vol}_n (B'').

 

1. What is Proposition 6.1.2 in D&K's Multidimensional Real Analysis II (Integration)?

Proposition 6.1.2 in D&K's Multidimensional Real Analysis II (Integration) states that if a function is integrable on a bounded set, then its integral over that set is equal to the limit of its integral over increasingly smaller subsets of that set.

2. How is Proposition 6.1.2 useful in real analysis?

Proposition 6.1.2 is useful in real analysis because it provides a way to calculate the integral of a function over a bounded set by breaking it down into smaller, more manageable subsets. This allows for more efficient and accurate calculations in multidimensional integration problems.

3. Can Proposition 6.1.2 be extended to unbounded sets?

Yes, Proposition 6.1.2 can be extended to unbounded sets. In this case, the integral over the unbounded set is defined as the limit of the integral over increasingly larger subsets of the set.

4. Are there any assumptions or conditions for Proposition 6.1.2 to hold?

Yes, there are some assumptions and conditions for Proposition 6.1.2 to hold. The function must be integrable on the bounded set, and the subsets used in the limit must have a finite measure. Additionally, the function must be continuous at almost every point in the set.

5. Can Proposition 6.1.2 be applied to functions with singularities?

No, Proposition 6.1.2 cannot be applied to functions with singularities. This is because the function must be continuous at almost every point in the set for the proposition to hold, and singularities are not continuous at those points. In this case, other integration techniques must be used.