Commutative diagram conventions

I'm used to seeing commutative diagrams where the vertices are mathematical objects and the edges (arrows) are mappings between them. Can the diagram ( from the interesting article https://people.reed.edu/~jerry/332/25jordan.pdf ) in the attached photo be interpreted that way?

In the article:

##k[x]## is the ring of polynomials over a field k.

##V## is a vector space.

##T## is a linear transformation on ##V##



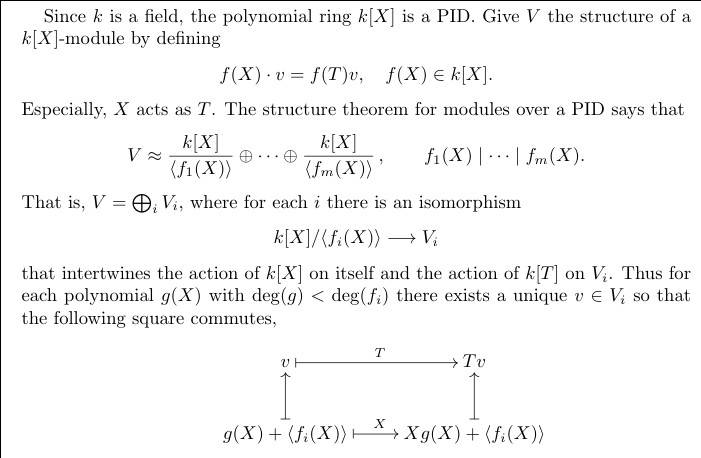
I understand ##T## and ##X## as maps, but do the vertical arrows go from a map to the argument of a map?

The arrows (all of them, vertical and horizontal) go from element to element. Their LaTeX code is \mapsto.

I'll understand the vertical arrow on the left this way: There is an (unnamed) isomorphism mapping ##V## to a direct sum of quotient modules. So ##g(x) + <f_i(X)>## is one element of that direct sum and it is in the coset of the ##<f_i(X)>##. So the unnamed isomorphism maps an element of the direct sum to a vector ##v## in ##V##.

the visual clue to what fresh and Stephen are saying is that the arrows have little tails at the beginning which are perpendicular to the arrow.