Differential structure of the group of automorphism of a Lie group

I am working on this



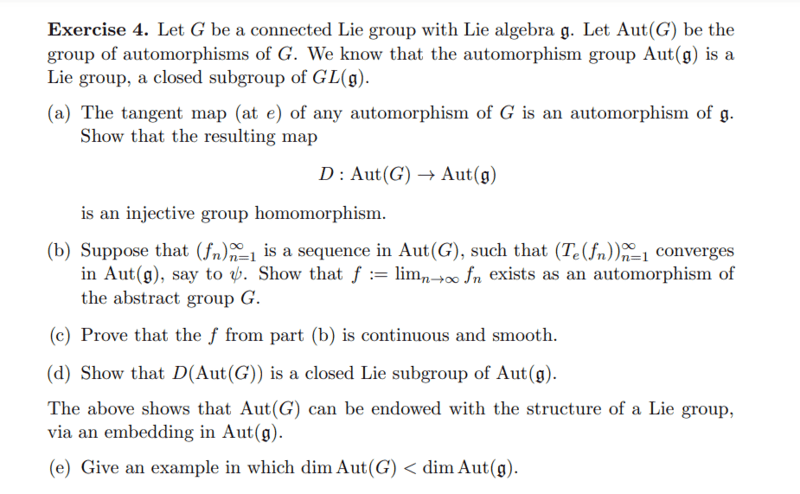
I am having trouble with b and c:

b) Suppose ##(f_n)_{n=1}^{\infty}## is a sequence in ##Aut(G)##, such that ##(T_e(f_n))_{n=1}^{\infty} \to \psi## converges in ##Aut(\mathfrak g)##

I want to show that ## f := \lim_{n\to \infty} f_n## exists as an continuous automorphism of the abstract group ##G##

First of all the ##f_n## are smooth, because they are in Aut(G), so they are Lie group isomorphisms, and therefore smooth by definition

Then by a known property of the exp:

##f_n(\exp(X))=\exp(T_ef_n X), \forall X \in T_eG## taking the limit yields:

##\lim_{n\to \infty} f_n(\exp(X))=\exp(\psi(X))\in G, \forall X \in T_eG##

##\lim_{n\to \infty} f_n(g)=\exp(\psi(X))=:f(g)\in G, \forall g## in a nbhd of the identity

I know that the image of the exponential map generates the connected component of the identity ##G_e##,and by connectedness this coincides with G so:
##G=G_e=<(T_eG)>=<\exp(\psi(T_eG))>=<\exp(T_eG)>##

This means that convergence is actually valid in the whole group, becuase if I have convergence in the generating set, I must have convergence in the generated set.

I hope this is correct,if not please tell me. Still I have to prove that it is bijective and a group homomorphism. I am not sure how to do this

b) How do I argue from here that ##f## is continuous? First I thought it was automatic, but then I recalled from analysis that pointwise convergence of a sequence of functions does not imply continuity. So I am clueless about how to proceed here as well