Automotive Alignment Theory - DIY Help

It seems that "most" stock sedans do not have adjustable caster, camber and turning angle, provisions are only provided to adjust toe only. I'm trying to find a way to DIY this at home, if this is possible, and had some questions on the theory behind it.

For my car, the stated toe specification for the front is "Front toe-in 0 +/- 2 mm (0+/-0.08 in)", from the service information from the manufacturer.

I see that there are toe plates, that you can purchase to attempt to DIY an alignment.

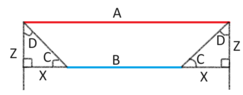
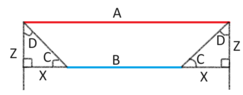
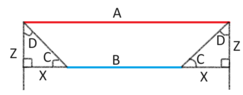
The basic theory in using this tool is that you place the plate up against the wheel, and take two measurements on either side.

I then subtract the length of line A vs the length of line B. I believe this term is called "Total Toe"?

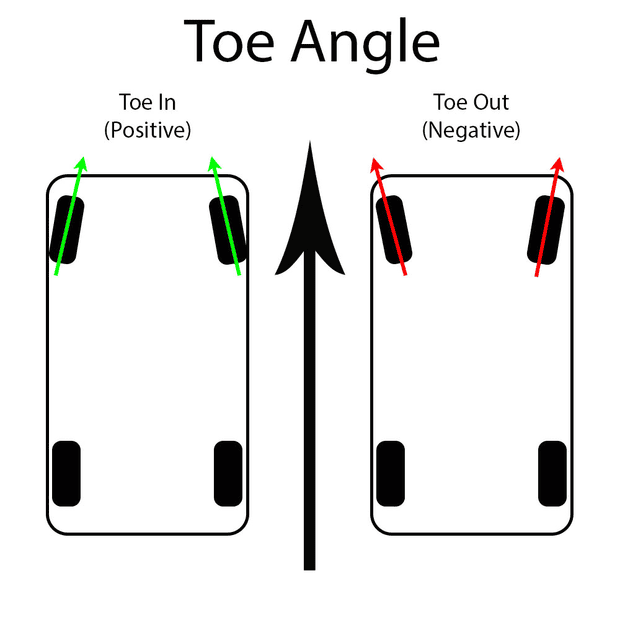
If( A - B > 0 ){
Toe Out
}
If( A - B < 0 ){
Toe In
}
if( A - B = 0 ){
Neutral Toe
}

This is pretty simple and straight forward.

However is the specified toe "Front toe-in 0 +/- 2 mm (0+/-0.08 in)" the "Total Toe" or the toe for each tire? If this is the toe for each tire, I don't see how to make this adjustment.

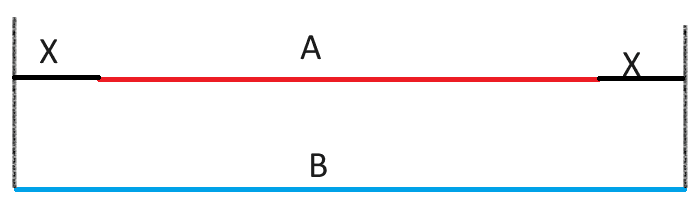
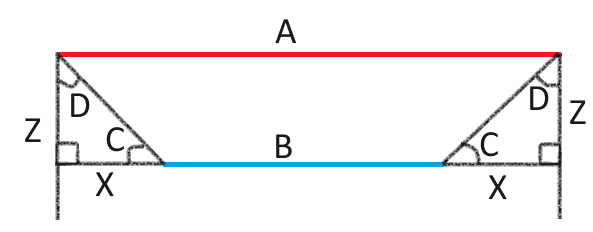
I'm not sure that I could just "assume" equal toe of each tire before adjustment. What I mean by this is that the difference between line segment lengths A and B are split evenly on either side of the line segment. In this rough sketch, I labeled both sides "X", indicating that I'm intending them to be the same length. Where abs(A - B)/2 = X


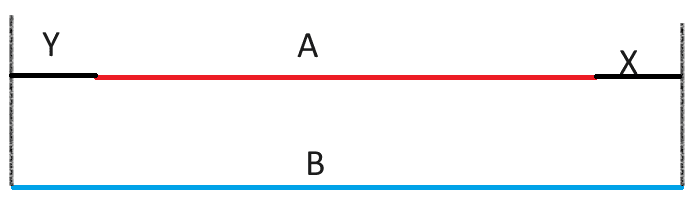
When in reality, I don't see why the difference has to be necessarily equal to each other and I can have this situation, where abs(A - B) gives me some variable Z that is equal to X + Y, and X =/= Y

If I could "assume" that the difference is split on either side evenly (which I doubt), I could see how I could do some simple trigonometry to find various angles. Given that the diameter of the tire, Z, is known and specified by the manufacturer of the tire.

In the toe in situation, which angle is the "toe of the tire" C or D?

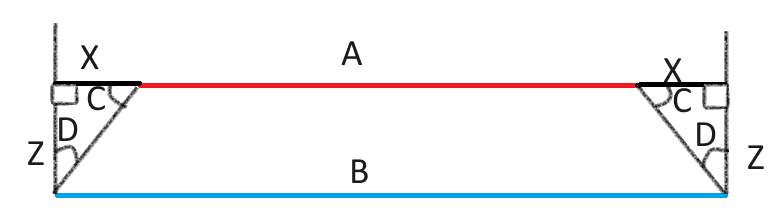
And in the toe out case, which angle is the "toe of the tire" C or D? Exaggerated to allow myself easier time drawing the picture, but you get the point.



I know how important having a "flat ground" is when doing alignments, but if all your concerned about is the toe, and toe plates is an ok way of calculating toe, then I don't see how working on a driveway that only "appears to be flat" but not may be perfectly flat would matter in measuring the the lengths of line segments A or B. Does the ground really need to be perfectly flat when all I'm worried about is toe?

I know that using these toe plates, does not account for variation in wall thickness of the tire or bulges. But when I have done alignments on an actual alignment rack, the sensors get mounted onto the tires, with arms the clamp down on the outside of the tires. It seems that this is not really something to worry about? I mean you could always remove the tire, brake caliper and rotor, and be left with nothing but the hub. But then you have to consider runout of the wheel hub. It seems like whatever you use to take the measurement is going to have some variation.

I see some manufacturers want you to have a full tank of gas, and alignment racks request that you measure the weight for the amount of "junk" that is in the persons car (provide an estimate), or remove it. Why is this? To me it would make more sense to perform an alignment on an alignment rack with the "normal" amount of weight that is the vehicle. If that means having 100 pounds of "junk", and 400 pounds of people, and a half tank of gas, then why even prompt the technician for this information, or to remove the "junk". Or maybe the owner keeps their car spotless and empty, and only they are in the vehicle. I don't know it just seems odd to me to account for the weight of stuff in the vehicle, as this constantly changes. I get that as you add more weight to the vehicle, the springs will compress, and ride height will decrease, this could effect some of the angles. So then wouldn't you just perform the alignment with whatever is the most common payload for the vehicle, in which case you would just perform the alignment with how the car came to the shop, if that means a spotless car, or a car with 100 pounds of "junk" in it, or even preforming the alignment the customer in the car!

Lastly, I was wondering if you can make adjustments to the outer tie rods and measurements, with the car up in the air on a lift? I do not see how having the car up in the air would change the lengths of line length A and B. Some cars are very low to the ground, and moving the outer tie rods and re-measuring is impossible with the car fully on the ground. It would be easier to jack it up a little bit, or have it on a lift and the suspension unloaded.

Thanks for any help in understanding toe alignment theory and nomenclature.

I've watched a DIY mechanic adjust toe, and done it once myself, both on a rear wheel drive car. The procedure may differ on front wheel drive.

1) match tire pressures, front tires should be same, back tires should be same

2) find an empty parking lot

3) while driving slowly

4) raise front of car until tires can rotate freely SEE NOTE BELOW

5) for each tire, while manually spinning tire, apply a chalk mark around the approximate middle of tire (you will have to rest your hand on a block to keep it steady)

6) while spinning tire, use a screwdriver blade or similiar to scrape a narrow line thru the chalk line. this gives a reference line for the toe measurements (again, you will need a hand rest)

7) put the steering wheel in the central/neutral position

8) measure distance between marked center lines of tires at the front-most part of tires. Write down the measurement!

9) measure distance between marked center lines of tires at the rear-most part of tires. Write down the measurement!

10) subtract the two measurements. the result should be within the specifications.

11) Lower front end so tires are bearing weight. Re-check toe measurements. If toe is out-of-spec, find a professional that can do it right! SEE NOTE BELOW

12) repeat the empty parking lot, hands free test for straight ahead tracking with hands-off steering wheel

NOTE 1:

YoshiMoshi said:

However is the specified toe "Front toe-in 0 +/- 2 mm (0+/-0.08 in)" the "Total Toe" or the toe for each tire?

First, I hate toe measured as a distance. It is very confusing as it is an angle. If you can find the spec as an angle value it is much clearer, otherwise convert it.

The "toe" is actually a measure of the "total toe" between two wheels on the same axle as being the difference between the centerlines of the tires measured at the rear edge of the tires minus the one measured at the front edge of the tires. So the toe for each tire requesting a total toe ##±2## mm on a ##645## mm tire diameter would be:
$$\sin^{-1}\left(\frac{±1}{645}\right) = ±0.09°$$

YoshiMoshi said:

When in reality, I don't see why the difference has to be necessarily equal to each other and I can have this situation, where abs(A - B) gives me some variable Z that is equal to X + Y, and X =/= Y

Setting the toe always begins with the rear axle. The rear toe will determine the thrust angle. The thrust angle is the angle between the car centerline and the thrust line.


Whether the rear toe is adjustable or not, the thrust angle will most likely not be 0°. You have to set your front toe according to that thrust angle otherwise, you will end up with what is called steer ahead, or how much you have to steer your front wheels to drive in a straight line.

YoshiMoshi said:

In the toe in situation, which angle is the "toe of the tire" C or D?

And in the toe out case, which angle is the "toe of the tire" C or D?

Always D. It is the angle with respect to the centerline for the rear and should be with respect to the thrust line for the front. The value measured is always toe-in, thus toe-out values are always negative.

YoshiMoshi said:

Does the ground really need to be perfectly flat when all I'm worried about is toe?

This and the rest of your questions about weight are to make sure the suspension positions are where they are supposed to be for the reference measurements. These specifications have been made at some reference points as they vary a lot based on where the wheels are situated with respect to the vehicle body. What matters is that you are where the manufacturer wants you to be. This is how the car was tested, with this particular specified setup.

One could argue that if you are always driving your car overloaded, some other specifications would be better. First, the manufacturer would not back up using their vehicle other than the way they were intended to be used. Second, one could argue that - if you whish to always run overloaded - increasing your spring rate to reset your correct ride height is a better solution than trying to find new alignment specs at the wrong ride height. Remember that alignment is not static and changes continuously, and not linearly, with ride height.

YoshiMoshi said:

I do not see how having the car up in the air would change the lengths of line length A and B.

Not only toe will change, but also the camber and wheel track will change too. A and B will most likely be different and the setting will not be done at the correct ride height.

The way I have done it in the past was by using two reference lines, one on each side of the car. I've seen two wood beams mounted on cans but I simply used two strings set at the axle height.

You can easily set those reference lines parallel by measuring the same distance at the front & rear ends of the car. It may not be parallel to the vehicle centerline though, but it is easy to find the angle between your reference lines and your centerline (The line going through the middle point of your front & rear tracks).

Then you can measure the distance from these reference lines to the rim edges of each wheel. With these measurements, you can find the "toe" for each wheel with respect to your reference lines. You can correct these angles to find the actual toe angles with respect to your centerline. You also now know the angle of your thrust line.

This is a lot of manual labor and needs some intensive computations (An Excel sheet seems appropriate to do the calculations) but it basically costs nothing in hi-tech tooling.

Hey thanks, I appreciate the help in understanding this.

jack action said:

The "toe" is actually a measure of the "total toe" between two wheels on the same axle as being the difference between the centerlines of the tires measured at the rear edge of the tires minus the one measured at the front edge of the tires. So the toe for each tire requesting a total toe ##±2## mm on a ##645## mm tire diameter would be:
$$\sin^{-1}\left(\frac{±1}{645}\right) = ±0.09°$$

So it sounds like a manufacturers specified "toe" is what some call "total toe", and that the definition that is described for toe, appears to be what toe plate tools measure.

However, this whole idea of a thrust angle, is a new idea to me, but you explained it well, and makes me question a few things with regards to front toe. It's good to learn. Sounds like rear toe needs to be adjusted first, otherwise, you cannot measure front toe.

jack action said:

Setting the toe always begins with the rear axle. The rear toe will determine the thrust angle. The thrust angle is the angle between the car centerline and the thrust line.

View attachment 334862
View attachment 334863​

Whether the rear toe is adjustable or not, the thrust angle will most likely not be 0°. You have to set your front toe according to that thrust angle otherwise, you will end up with what is called steer ahead, or how much you have to steer your front wheels to drive in a straight line.

View attachment 334864​

Always D. It is the angle with respect to the centerline for the rear and should be with respect to the thrust line for the front. The value measured is always toe-in, thus toe-out values are always negative.

For my particular car, rear toe is adjustable and is "Rear toe-in: 2 +/- 2 mm (0.08 +/- 0.08 in)". My particular car, does not have a rear axle, just rolls. But I think the same concept applies.

So if I were to do this on my own, I would measure toe using toe plates, which seems to be an accurate technique, as accurate as the tape measure.

Making a corrections to my previous picture

• Putting the Variable Z where it's supposed to be as the diameter of the tire
• Labeling DL, CL, CR, and DR, for "C Left", "C Right" etc.

Let's take the rear toe in case.

• I measure and observe the length of line segment A.
• I measure and observe the length of line segment B.
• I know the diameter of the tire Z, it's specified by the manufacturer
• I know that in order to alignment the rear toe, the length of line segment B should larger the length the length of line segment A.

After reading through your explanation a few times and looking at the rough sketch, I still seem a bit confused. For a total toe of +/- 2 mm on a 645 mm tire diameter, why would you calculate the toe of each tire as:

$$\sin^{-1}\left(\frac{±1}{645}\right) = ±0.09°$$

It seems like this would assume that the specified toe of +/- 2 mm would be split between each tire evenly, meaning X would be equal to Y in my picture. If this assumption is accurate, than the calculation above would seem to be correct. Can this assumption be made though, and if so why? Is it just the ideal case for the toe to be split evenly? To me it would make sense to do this, but I'm not sure if it can just be assumed that this is the case? I can measure A and B, but won't be able to know if the difference between A and B is split evenly or not.

Sorry if I'm being a pain, I just want to make sure I have a true understanding of this. The technicians performing the alignment, most likely don't know the math behind it, and they don't need, they just need to get the angles in the green for the customer. But as a result, the math is a bit hard to understand.

Thanks for the explanation on the weight. Just seemed odd to me when software on alignment racks take into consideration the weight of one gallon of gasoline. Making me believe that when you go in for an alignment, for best results, your car should be as empty as possible, but should still have the spare in it.

So the suspension needs to be fully loaded and the car fully on the ground in order to get accurate toe measurements. This makes me thing that this would be pretty difficult to DIY. Your car would have to have to have a high ride height at stock, to crawl underneath the car and adjust the outer tie rods for example in the front. For example, a modern day Honda Civic, forget it. There's not enough space underneath the car to attempt to crawl under and adjust the outer tie rods.

Also I have heard some technicians modify a setting on the alignment racks to specify angles in only tenths of a degree. Because going out to 100th of a degree, could lead to customers questioning why one side is different than the other (suggesting that perhaps having it split evenly is ideal), by a few hundredths of a degree. That such a small difference isn't going to matter, for a car going max 75 MPH in the worse case situation.

Production automobiles use toe-in and have adjustments for caster and camber on the front and rear wheel assemblies. Toe-out is used on race cars. The theory of toe-in is the wheels want to move to a toe-out position under speed. Toe-in can lead to higher rolling resistance, which may cause less fuel mileage.

Why toe-in or toe-out?

A wheel alignment with zero toe will wander and feel unstable. In a race car the car will be too darty. So we induce a small amount of drag to keep the car stable. Production cars use front end components mounted in rubber or synthetic isolators in control arms, A-arms, ARB, to reduce vibration and add to drive comfort. Race cats use steel bushings to accurately maintain alignment and drivers do not care they have steering wheel vibration.

Why alignment? Bad wheel alignment causes -

Uneven tread wear

Vehicle pulling to the left or right

Your steering wheel is off center when driving straight

Steering wheel vibration

Caster angle helps balance steering, stability, and cornering. Camber is the inward or outward angle of the tire when viewed from the front of the vehicle

Caster, camber and toe have been easily adjusted in the garage by competent mechanics with very simple tools. Been that way for years.

YoshiMoshi said:

Can this assumption be made though, and if so why? Is it just the ideal case for the toe to be split evenly? To me it would make sense to do this, but I'm not sure if it can just be assumed that this is the case?

If the toe is not even on both sides, the axle will steer. Setting up toe to a wheel introduces a lateral force on the tire. If both sides have equal and opposite forces, the vehicle goes in a straight line; if not it steers.

Imagine having the requirement of 0° toe (A = B) but one wheel has 10° toe-in and the other 10° toe-out. You have both wheels steered 10° in one direction and you will have to turn them back 10° with your steering wheel to drive the vehicle in a straight line. This causes two problems:

1. The steering wheel is not centered anymore. You may have a steering wheel branch blocking your view of the dashboard;
2. A steering system may have a central position as well, one that is critical:

https://www.motortrend.com/how-to/1904-we-test-four-different-ratio-saginaw-power-steering-boxes/ said:

Get Centered
With most Saginaw power steering boxes, the input shaft is connected to the steering column through a rag joint that allows for some flex in the connection between the two shafts. The “on center” position is critical during installation, something that very few people fully understand from our small sample survey of enthusiasts. What this means is that the steering gear is simply installed in the mid-point of the steering gear to allow the same amount of steering input in both directions. Most importantly, however, is the fact that this midpoint also has a tighter tolerance between the piston rack gear and sector shaft pinion. This eliminates any play when driving in a straight line. This is different from simply aligning the steering wheel during setup.

YoshiMoshi said:

This makes me thing that this would be pretty difficult to DIY.

It can be. Between each measurement, you should push down on the car a few times and move the car backward and forward to settle the suspension. Just by lifting the tire off the ground and putting it back down, the lateral friction may prevent the tire from getting to its natural position (affecting camber measurement). As mentioned by @Tom.G , alignment guys usually have the car resting on turntables which helps prevent this when adjusting the toe (even without lifting the car).

Following is a setup to do an alignment with stands and turntables. It requires lifting the vehicle 3 feet high but you could have lower stands, just enough to clear the ground:



How difficult it is depends on how fussy you are.

You can easily adjust toe by your self. You have to lift the front end up to adjust the tie rod ends and once the tires are back on the ground you "jounce" the suspension back to normal.
chap caster camber gage but this usually is way over the average persons tech.



https://www.amazon.com/dp/B07LG1TMTZ/?tag=pfamazon01-20

https://www.amazon.com/dp/B001NNFZ2Q/?tag=pfamazon01-20

or make toe bar your self

Hey thanks! So I understand now that after the alignment is performed, the toe angle on each tire should be the same. This allows the vehicle to travel in a straight line. I think I understand the math now, and the angle. I understand this. However, is it safe to assume that my car current (before an alignment), that the toe on each tire is the same. Assuming that I hadn't gotten into an accident, or replaced any suspension components. I don't know if the rate of wear in ball joints, ride height lowering with age/time/wear, etc. would be the same for the left and right tires? This seems like a reasonable assumption to make. I just want to make sure that this indeed the assumption that is being made, and if it is a valid one? If this is the case, then I see how adjusting toe can work, easily. If this is not the case, I'm a bit confused on how you can DIY this.

If this is indeed the case, if I take for example the front toe, with front steering. Toe is adjusted via outer tie rods. So I could mark the outer tie rod nut in some way with a paint marker corresponding to a point on the nut that rotates with the inner tie rod, and put numbers on the points of the nut. Something like this.

As I rotate the nut, I can keep track of how many revolutions I do of the inner tie rod. What I do to one side, I should do to the other as well, because we want the same toe on each side.

1) Take measurement with toe plates, see if within the specification
2) Lift up the vehicle
3) brake loose the nut on the inner tie, while making sure to hold the inner tie rod to prevent it from rotating; do this to both sides.
4) Move the nut up the inner tie rod a few threads to allow for rotation of the inner tie rod to occur; do this to both sides
5) Rotate the inner tie rod and the nut will move with it. Keep track of how many many revolutions (including any partial of a revolution) you do by referencing the line you made on the outer tie rod and the numbers you wrote on the nut; apply the same number of rotations (including any partial of a revolution) to the other side
6) Retighten the nut to ensure no additional rotation
7) Put car back on ground
8) bounce on the suspension a few times
9) Repeat steps 1-8 as needed until within specification

It seems most measuring tapes in the states are marked with an inch scale, with a resolution of 1/32 of an inch.

So if we talking about:
Front toe-in 0 +/- 2 mm (0 +/- 0.08 in);
Rear toe-in: 2 +/- 2 mm (0.08 +/- 0.08 in);

1 tick mark = 2/64 in = 1/32 in = 0.03125 in
2 tick mark = 4/64 in = 2/32 in = 1/16 in = = 0.0625 in
2.5 tick mark = 5/64 in = 0.078125 in
3 tick mark = 6/64 in = 3/32 in = 0.09375 in
4 tick mark = 8/64 in = 4/32 in = 2/16 in = 1/8 in = 0.125 in
5 tick mark = 10/64 in = 5/32 in = 0.15625 in
5.5 tick mark = 11/64 in = 0.171875 in
6 tick mark = 12/64 in = 6/32 in = 3/16 in = 0.1875 in

When I measure A and B in the front, I need B to be larger than A by no more than approximately 2.5 tick marks on a typical measuring tape with a resolution of 1/32 of an inch.

When I measure A and B in the rear, I need B to be larger than A by no more than approximately 5.5 tick marks on a typical measuring tape with a resolution of 1/32 of an inch.

Looking at how small the displacement is of 1/32 at a tape measure in real life, I think even the thickness of the toe plate would need to be taken into consideration, as it might be thicker than 1/32 of an inch, and would be good to take the measurement on the inside of the toe plate, so the thickness of the toe plate doesn't throw the measurement off.

YoshiMoshi said:

However, is it safe to assume that my car current (before an alignment), that the toe on each tire is the same.

Not necessarily. Many roads are crowned to allow surface water to run off into a gutter and not create puddles in the roadway.

Unless you are driving in the middle of the road, you are constantly driving on a hillside. This cause a slight weight shift to one side and, at high mileage, a bit more wear on tires/suspension.

Cheers,
Tom

Thank you everyone!

I seem to have a good understanding now. The only way it seems that this can be done via DIY is what people have mentioned with reference lines, blocks of wood, etc.. The rear of the vehicle, toe is adjusted with respect to the centerline of the axle. I would need two lines parallel to this centerline, or account for the angle difference. Than take two measurements from these parallel lines to the rim.

I'm not really sure what are the use of toe plates, except to check for total toe. But sense we don't know the toe of each tire. Doesn't seem to useful, other than to check to see if the total toe is off and you need an alignment. But even if the total toe is correct, you could still need an alignment, because the toe for each tire may not be equal. Is there a more useful DIY tool to check the toe of an individual tire, other trying to create your own reference lines and taking measurements from them?

A similar process would be required for the front, except instead of the centerline, my measurements would be with regards to the thrust angle line. In my case for the front, I would want the two measurements to be within 1 mm of each other for each wheel. Not only that I would want the same difference for the other tie on the front axle. Can this be accurately done via DIY? It would seem that even variation in the thickness of what I'm using for reference lines to the rim (if that is what I was measuring to), a block of wood, or metal plate, or a piece of string, could throw off this measurement. 1 mm is roughly the thickness of 10 sheets of paper or a dime. I could always use cheap harbor freight micrometer, and use string up against the rim. Just not sure how accurate I can be on the order of magnitude of 1 mm. Or I guess technically even smaller, 1/100 of an inch or about 2.5 sheets of paper thick.

If with a micrometer I measure 0.01 inches for the front of the tire, and 0.04 for the rear of the tire. I see that I'm within specification of slightly less than 0.04 inch difference. I would then adjust toe on the other side so that I can the same measurements to produce the same toe angle.

YoshiMoshi said:

However, is it safe to assume that my car current (before an alignment), that the toe on each tire is the same.

If that wasn't the case, your steering wheel should be off-center when driving in a straight line on a flat surface.

A better question is "Is your toe-in alignment off?" Here are the symptoms to look for:

https://tiregrades.com/tire-maintenance/alignment/too-much-toe-in-alignment/ said:

Too Much Toe-In Alignment
Too much toe-in alignment in a vehicle can cause symptoms like uneven tire wear, especially along the inner edges, off-center steering wheel position, reduced straight-line stability, and decreased fuel efficiency. It is primarily caused by worn or damaged steering or suspension components, or by wheel impact events like hitting a curb.

If you don't have any of these symptoms, you probably don't have to play with the toe at all.

YoshiMoshi said:

Can this be accurately done via DIY?

How good an alignment is depends on your expectations. If the specs call for 2mm and the manufacturer says you can go up to 4mm, if you set it to 6mm, you probably won't notice anything. Again, it depends a lot on how fussy you can be.

YoshiMoshi said:

When I measure A and B in the front, I need B to be larger than A by no more than approximately 2.5 tick marks on a typical measuring tape with a resolution of 1/32 of an inch.

The spec is 0mm for the front. Ideally, A should be equal to B. But you are allowed to go from -2mm to 2mm, thus A or B can be the longer measurement.

Just to make sure I'm understanding here. I could in theory use toe plates and align the front pretty easy to ensure that have zero toe. The two measurements, A and B would just need to be equal. I could get this with a typical non-specialty tape measure from the hardware store that is accurate to within 1/32 of an inch. Or could get highly accurate tape measures that are a bit more expensive that have 1/50, 1/64, or even 1/100 of an inch graduation marks.

But, if I'm understanding correctly, while I could get zero toe with a high accuracy for the front axle, I will not be able to confirm if that zero toe is indeed really zero along the thrust angle axis, or some other unknown axis. Therefore it wouldn't really be accurate.

Just want to make sure I have the correct understanding here.

look, Zero toe will make the car darty and you will not like it. Too much toe in or toe out will wear tires. set it up to factory toe specs and forget about it. Worst case is bad tire wear, less miles per gallon.

YoshiMoshi said:

I will not be able to confirm if that zero toe is indeed really zero along the thrust angle axis,

If your steering is centered while measuring the correct A and B, if it is not with respect to the thrust axis, your steering wheel will be off-center when driving in a straight line (i.e. you will notice). In such a case, you have to move both wheels in the same direction while keeping A and B the same until your steering wheel is centered both while measuring and when driving in a straight line.

But that won't tell you if your thrust axis is parallel to your vehicle's centerline. If it is not, your vehicle will drive "sideways" in a straight line, but that should be rather unnoticeable. (And if it is, you surely need to correct your thrust axis.)

Imagine having your steering wheel centered and setting the appropriate total toe while both wheels obviously have a 45° angle pointing to the left. When you will want to drive in a straight line, you will necessarily have to turn your steering wheel to the right a few turns. Not only your steering will be off-center but the total toe will most likely not be the same anymore.

Ranger Mike said:

Zero toe will make the car darty and you will not like it.

In his case, zero is the spec.

So if I'm understanding correctly. Setting toe to specification using DIY equipment is nearly impossible. However if I replace part of a suspension, I can get it back to the toe it was before part replacement? Not saying that the previous alignment was correct, but if I recently had an alignment done, haven't gotten into an accident, and don't have any abnormal tire wear, should be ok?

Would below work to get back to the previous alignment after replacing part? I don't see why not.

1) Using toe plates, take your two measurements, A1 and B1 of front tires.
2) Replace outer tie rod on LH side.
3) Lower car back onto ground and take two measurements using toe plates, A2 and B2.
4) Adjust outer tie rod on LH side.
5) Repeat steps 3 and 4 until A2 = A1 and B2 = B1.

Seems pretty simple to me and straight forward. This would get you within 1/32 of an inch using basic tape measures, and could get even more accurate using specialty ones that more "tic marks".

YoshiMoshi said:

Would below work to get back to the previous alignment after replacing part? I don't see why not.

1) Using toe plates, take your two measurements, A1 and B1 of front tires.
2) Replace outer tie rod on LH side.
3) Lower car back onto ground and take two measurements using toe plates, A2 and B2.
4) Adjust outer tie rod on LH side.
5) Repeat steps 3 and 4 until A2 = A1 and B2 = B1.

If you replace a tie rod, it is necessarily because it has excessive play or is damaged.

• Play: Measurements are impossible to take;
• Damaged: Alignment is most likely wrong.

Thus A1 & B1 should be wrong every time.

But even assuming alignment is good and you only are changing your tie rod because you don't like the color(!) or something, all you have to do is measure the distance between the outer tie rod pivot point and the inner tie rod pivot point (or maybe just the boot end on a steering rack) and reinstall with the same measurement. I doubt you would do much better than that with your toe plates; it would only be for double-checking purposes.

YoshiMoshi said:

Setting toe to specification using DIY equipment is nearly impossible.

It is not. I once took the frame of my car to restore it, put brand new steering & suspension parts, and aligned it myself as per post #3. I never had any problem with the alignment.

Here's an article titled DIY Wheel Alignment - It’s Easier Than You Think! It is written with week-end racers in mind, because going for a professional alignment for every little change you want to make to your setup, between every race, would be crazy. On page 2 there is an explanation about the "string" method, similar to what I did. You are really overthinking this.

I can see how you can DIY an alignment, if the specified rear toe is 0. You can then easily do both the rear and front toe using the string method mentioned above. For the string method, the string is a line parallel to the centerline, and with zero rear toe, thrust line = centerline. So the string method can be used.

But in the case for this car the ideal rear toe is 2 mm

Front toe-in 0 +/- 2 mm (0 +/- 0.08 in);
Rear toe-in: 2 +/- 2 mm (0.08 +/- 0.08 in);
Front/Rear Tire Size: P225/50R17

ideal toe for one rear tire = arcsin( (( 2 mm)/2)/(658 mm) ) ~ 0.0870756863 degrees

This is incredibly small. It's possible that with the string method, that the string may be off 0.09 degrees from true centerline? But assuming that the string is perfectly parallel to the centerline.

sin( ideal toe for one rear tire ) = A / 658 mm
658 mm * sin ( arcsin( (( 2 mm)/2)/(658 mm) ) ) = 1 mm = 0.0393701 inches

So I would want side A to be slightly more than 1 graduation mark using a typical tape measure that you can get home depot with 1/32 inch scale. Or using a cheap digital micrometer from harbor freight.

So if I'm understanding this correctly the thrust angle is about 0.174151373 degrees.
I don't even know how I would go about aligning toe in the front, using the string method, were my reference line is 0.174151373 degrees off from true centerline to the passenger side (steering wheel on the left), or how I would go about approximating it.

I perfectly get how the string method can get you close if ideal toe in the rear is zero, but otherwise it's still a bit confusing to me.

I may be overthinking this. Hopefully someone can shed some light on this for me.

YoshiMoshi said:

So if I'm understanding this correctly the thrust angle is about 0.174151373 degrees.

You don't understand correctly: the ideal thrust angle is always zero, i.e parallel to the centerline (actually right on it).

First, you can set your rear toe to zero if that is what you wish, it is within the specs (between 0 mm & 2 mm on each wheel).

Second, if you take the measurements near the outside of the tires (assume you install your toe plates) it is still a ±1 mm difference allowed, i.e. you aim for 1 mm but it could be anything between 0 & 2 mm. It is not more difficult than measuring it from the inside between the tires where you aim at ±2 mm (on a, maybe, 1500 mm length) instead of ±1 mm (on a, maybe, 75 mm length).

Third, I'm not sure why you think having the rear toe at zero makes it easier, but here's how you can do it:

• You set two strings on each side with both string ends at equal distances (on the pipes in the picture above);
• You move the strings such that the centers of the wheels/axles are the same distance from the strings on both sides (the rear and front will most likely be different). Now both strings are parallel with the centerline of the axles;
• Note that your axles may not be perfectly perpendicular to that centerline. Even if the wheel center-to-center distance on each side is the same, both axles will be parallel to each other but not necessarily perpendicular to the centerline. Unless the car is badly damaged, this is unimportant;
• You set the toe at the rear with respect to the strings (i.e the centerline), such that the thrust line will necessarily be on the centerline;
• Then you set the front toe the same way to have the front axle going in the same direction.

That's it. Your alignment is done. Your four wheels will all point in the same direction (imagine zero toe everywhere to visualize it better).

Thanks for helping me understand. This topic is not explained very well in school. Just throw on the sensors on an alignment rack, and make the adjustments until green.

Front toe-in 0 +/- 2 mm (0 +/- 0.08 in);
Rear toe-in: 2 +/- 2 mm (0.08 +/- 0.08 in);
Front/Rear Tire Size: P225/50R17

I could have sworn somewhere in this thread I saw that some slight positive toe in in the rear was actually desired, because it helps keep the car moving straight ahead. But I can't seem to find it. But then I see in the post above that zero thrust angle is actually desired. If the rear toe is other than zero, you have a thrust line. I assumed that from the specifications 2 mm for the rear axle plus some tolerance, also suggested that a slight toe in was actually desirable as well, and the ideal rear total toe is actually 2 mm.

Rear Axle - Reference line is vehicle centerline
Front Axle - Reference line is thrust line (if rear toe is zero, thrust line = centerline)

Have to do rear first, to determine thrust line.

I fully get that the strings are a translation of the vehicle centerline vector, and how it can be used for the rear toe measurement, as rear toe is with respect to centerline.

But if rear toe is other than zero, the string (translation of vehicle centerline vector), is not of help for aligning the front as the thrust line is the reference line instead.

I think having zero rear toe is easier, because than the string method can be used because
String = translation of vehicle centerline vector = thrust line.

If I attempt to align the rear total toe to 2 mm with respect to the vehicle centerline (the string) as the reference line, then my thrust angle is non-zero. I then have to align the front toe with respect to the thrust line (which would be different then the centerline and is not the same thing as the string).

jack action said:

Whether the rear toe is adjustable or not, the thrust angle will most likely not be 0°. You have to set your front toe according to that thrust angle otherwise, you will end up with what is called steer ahead, or how much you have to steer your front wheels to drive in a straight line.

I think maybe I misunderstood this part? I was understanding this as the front toe is adjusted with respect to the thrust angle/line. If the rear toe is other than zero, the thrust line is not equal to the centerline (string), and you use the thrust line (not the string) as the reference line. If the rear toe is zero, thrust line is equal to the centerline (string), and you can use the string to make toe adjustments to the front and rear axles.

YoshiMoshi said:

if rear toe is zero, thrust line = centerline

I'm not sure what you don't understand about the thrust line but the proper statement would be: if the left rear toe angle is equal and opposite to the right rear toe angle, with respect to the centerline, thrust line = centerline.

For example (I'll use large numbers for clarity) see these values with respect to the centerline:

• left: 0° toe-in; right: 0° toe-in -> thrust line = centerline
• left: 5° toe-in; right: 5° toe-in -> thrust line = centerline
• left: 7° toe-in; right: 3° toe-in -> thrust line direction is 2° pointing right compare to centerline
• left: 10° toe-in; right: 0° toe-in -> thrust line direction is 5° pointing right compare to centerline
• left: 5° toe-in; right: 5° toe-out -> thrust line direction is 5° pointing right compare to centerline

Except for the first and last examples, they all have a 5° toe-in with respect to the thrust line. The first and last examples both have 0° toe with respect to the thrust line. By definition, the left side angle must be equal to the right side when measured from the thrust line. The axle follows the direction of the thrust line.

If you can adjust the rear toe, great, you set it up to correspond to the centerline. But if you cannot - assuming the thrust angle is within specs (i.e. no damage) - it is better to adjust your front toe along the thrust line rather than the centerline because this is the path your rear axle will follow in a straight line.

I truly admire your efforts displayed in this post.
You have no feed back loop to measure your efforts so you are going thru a mental masturbation for perfection that does not fit the real world conditions. I can absolutely tell you, Perfect setup in the shop does not equal real world race track performance.
It is real simple. You want the best alignment for your car on your daily drive on your road ways under your climate conditions. The factory settings are a compromise for the average purchaser of your car and are geared for the average conditions- worldwide. Car manufacturers do not know where the car will end up after purchase. Their settings are a base line. Country roads are much different than highway paved surfaces. Road surfaces are not universal and constant.
In racing ,we tune the chassis and tire contact patches to the track for the current racing conditions.

Buy a cheap tire pyrometer with the needle temperature sensor and measure the tire edges of the front tires after driving ten miles. If one side it hotter on the inside edge compared to the other side, adjust the toe accordingly. Then drive it.

If you really want to play around with the best alignment, then keep a good log book recording your adjustment and the results.