- #1
nateTheaweseome
- 14
- 2
- TL;DR Summary
- Calculated handle bar pulling force to be significantly lower than what I measured in real life; This is attached to 2 brake cables, each connected to one end of a gas piston (tension).
Hey all,
I'm either overthinking it or too tired but this problem should be an easy one to figure out but I'm stumped embarrassingly.
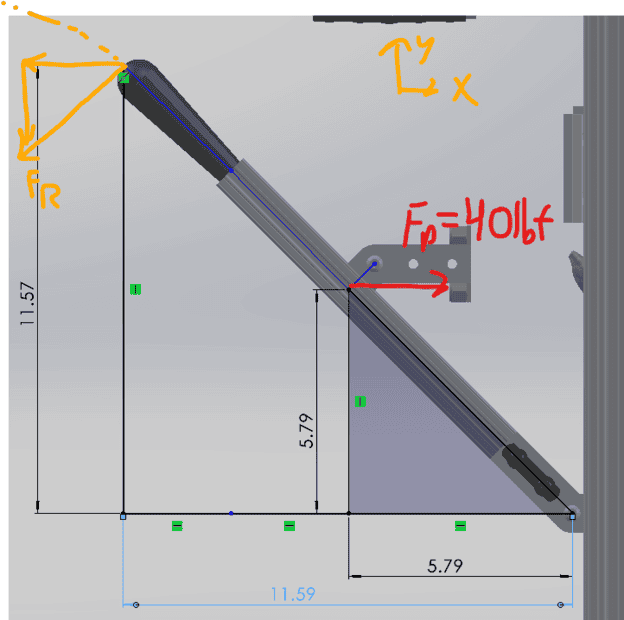
Say in the photo above is a lever arm. I'm trying to backtrack to calculate the force a user must input (yellow) against a force (red). It can pivot around the hinge, and when I calculate the Fr by summing moments around the pivot and solving for Fr, I get around 14lbf. This is designed so that Fp stays horizontal for the range of motion on the arm (not meant to go completely flat).
Now, this doesn't make sense because in real life, I measured this to be about 70lbf...Granted, several things could be wrong here:
1. I screwed up my moment equation (the 40lbf doesn't have a component in Y does it?)
2. I'm thinking this the wrong way
3. The Fp value is wildly wrong. It's a long story but TL;DR That force is coming from 2 different brake cables, attached to both ends of a tension gas piston (rated at 20lbf compression, 34lbf extension). The way I got 40lbf is that when the gas piston is not displaced, the force is around 20lbf, and multiply that by 2 since there's two brake cables attached to both ends of the piston, so the lever at the mounting point will see 40lbf. Now as I understand the compression force increases as displacement increases like a spring but even when I change the total Fp value to be 68lbf, it's no where near what I measured in real life.
I'm kinda stumped so any help would be great.
I'm either overthinking it or too tired but this problem should be an easy one to figure out but I'm stumped embarrassingly.
Say in the photo above is a lever arm. I'm trying to backtrack to calculate the force a user must input (yellow) against a force (red). It can pivot around the hinge, and when I calculate the Fr by summing moments around the pivot and solving for Fr, I get around 14lbf. This is designed so that Fp stays horizontal for the range of motion on the arm (not meant to go completely flat).
Now, this doesn't make sense because in real life, I measured this to be about 70lbf...Granted, several things could be wrong here:
1. I screwed up my moment equation (the 40lbf doesn't have a component in Y does it?)
2. I'm thinking this the wrong way
3. The Fp value is wildly wrong. It's a long story but TL;DR That force is coming from 2 different brake cables, attached to both ends of a tension gas piston (rated at 20lbf compression, 34lbf extension). The way I got 40lbf is that when the gas piston is not displaced, the force is around 20lbf, and multiply that by 2 since there's two brake cables attached to both ends of the piston, so the lever at the mounting point will see 40lbf. Now as I understand the compression force increases as displacement increases like a spring but even when I change the total Fp value to be 68lbf, it's no where near what I measured in real life.
I'm kinda stumped so any help would be great.