- #1
zenterix
- 423
- 60
- TL;DR Summary
- There is a surface shown in the book "Physical Chemistry" by Silbey, Alberty, and Bawendy that I would like to understand better.
Here is the figure I would like to understand
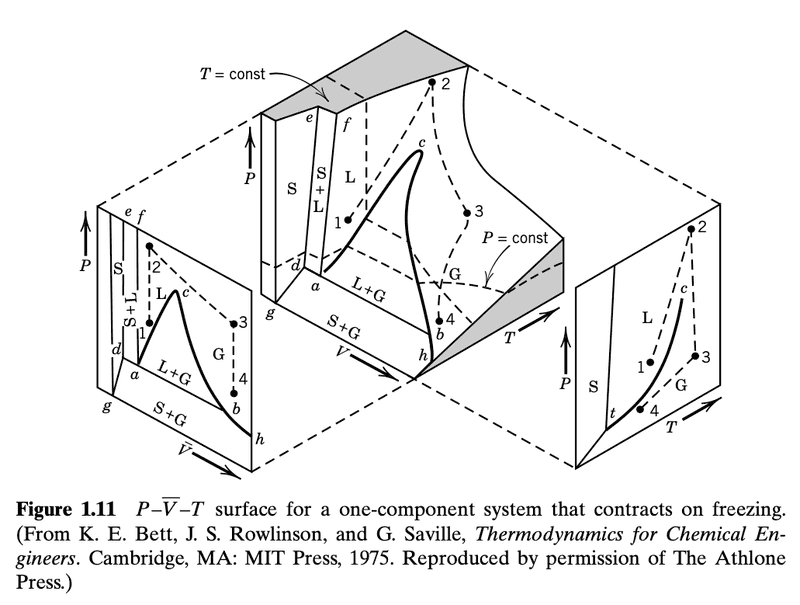
First of all, I don't see a specific surface. In the middle plot, I see what looks like the innards of a solid cube that has a large piece cut off.
There is also an arrow annotated as "T=const". It is not clear what this arrow is pointing to. I though it was the shaded plane but that doesn't seem correct. So, perhaps that small line segment on the shaded plane?
Furthermore, the book says that
and that
Also, consider the following quote
In figure 1.11, I guess this is denoted as just ##c##. I suppose that ##P_c## and ##T_c## are different for each volume and so for each ##P_c## and ##T_c## we have an associated ##V_c##.
First of all, I don't see a specific surface. In the middle plot, I see what looks like the innards of a solid cube that has a large piece cut off.
There is also an arrow annotated as "T=const". It is not clear what this arrow is pointing to. I though it was the shaded plane but that doesn't seem correct. So, perhaps that small line segment on the shaded plane?
Furthermore, the book says that
"the state of a pure substance is represented by a point in a Cartesian coordinate system with ##P, \bar{V}##, and ##T## plotted along the three axes. Each point on the surface of the 3D model in Fig. 1.11 described the state of a one-component system that contracts on freezing".
and that
"Projections of this surface on the ##P-\bar{V}## and ##P-T## planes are shown. There are three two-phase regions on the surface: S+G, L+G, and S+L. There three surfaces intersect at the triple point ##t## where vapor, liquid, and solid are in equilibrium".
Why is the slope dP/dT positive when substances contract on freezing?The projection of the three-dimensional surface on the P –T plane is shown to the right of the main diagram in Fig. 1.11. The vapor pressure curve goes from the triple point t to the critical point c (see Section 1.7). The sublimation pressure curve goes from the triple point t to absolute zero. The melting curve rises from the triple point. Most substances contract on freezing, and for them the slope dP/dT for the melting line is positive.
Also, consider the following quote
"For a pure substance there is a critical point ##(P_c , T_c)## at the end of the liquid–gas coexistence curve where the properties of the gas and liquid phases become so nearly alike that they can no longer be distinguished as separate phases. Thus, ##T_c## is the highest temperature at which condensation of a gas is possible, and ##P_c## is the highest pressure at which a liquid will boil when heated."
In figure 1.11, I guess this is denoted as just ##c##. I suppose that ##P_c## and ##T_c## are different for each volume and so for each ##P_c## and ##T_c## we have an associated ##V_c##.
Last edited: