walter lewin

A New Interpretation of Dr. Walter Lewin’s Paradox

/65 Comments/in /by
Estimated Read Time: 7 minute(s)
Common Topics: loop, meter, voltmeter, battery, resistor

Much has lately been said regarding this paradox which first appeared in one of W. Lewin’s MIT lecture series on ##{YouTube}^{(1)}##.  This lecture was recently critiqued by C. Mabilde in a second YouTube video and submitted as a  post in a PF ##{thread}^{(2)}##.  The latter cited the third source, that of K. T. McDonald of Princeton University, as support for Mabilde’s ##{presentation}^{(3)} {and}^{(4)}##.  Finally, Charles Link, PF Homework Helper, and Insight Author posted in Advisory Lounge Inner Circle (#1, May 25, 2018) on the same subject.  Furthermore, several other PF individuals (and probably others still)  have been involved in this topic.

I think a key concept, which none of the three sources mentions, is that there are two ##E## fields running around here.  One is the static field ##E_s## which by definition is conservative and the field lines of which begin and end on charges.  The second is the emf-induced field ##E_m## which is non-conservative in the sense that its circulation is non-zero.  ##E_m## can be created by a chemical battery, magnetic induction, the Seebeck effect, and others.

In the case of Faraday induction its circulation is Faraday’s  ## \frac {-d\phi} {dt} ##. The two fields cancel each other in any loop wire segment but only ##E_s## exists in the resistors.  (This statement assumes negligible resistor body lengths and zero-resistance wires).  The net ##E## field is anywhere and everywhere just ## E = E_s + E_m ##, algebraically summed.

A voltmeter reads the line integral of ##E_s##, not any part of ##E_m##.

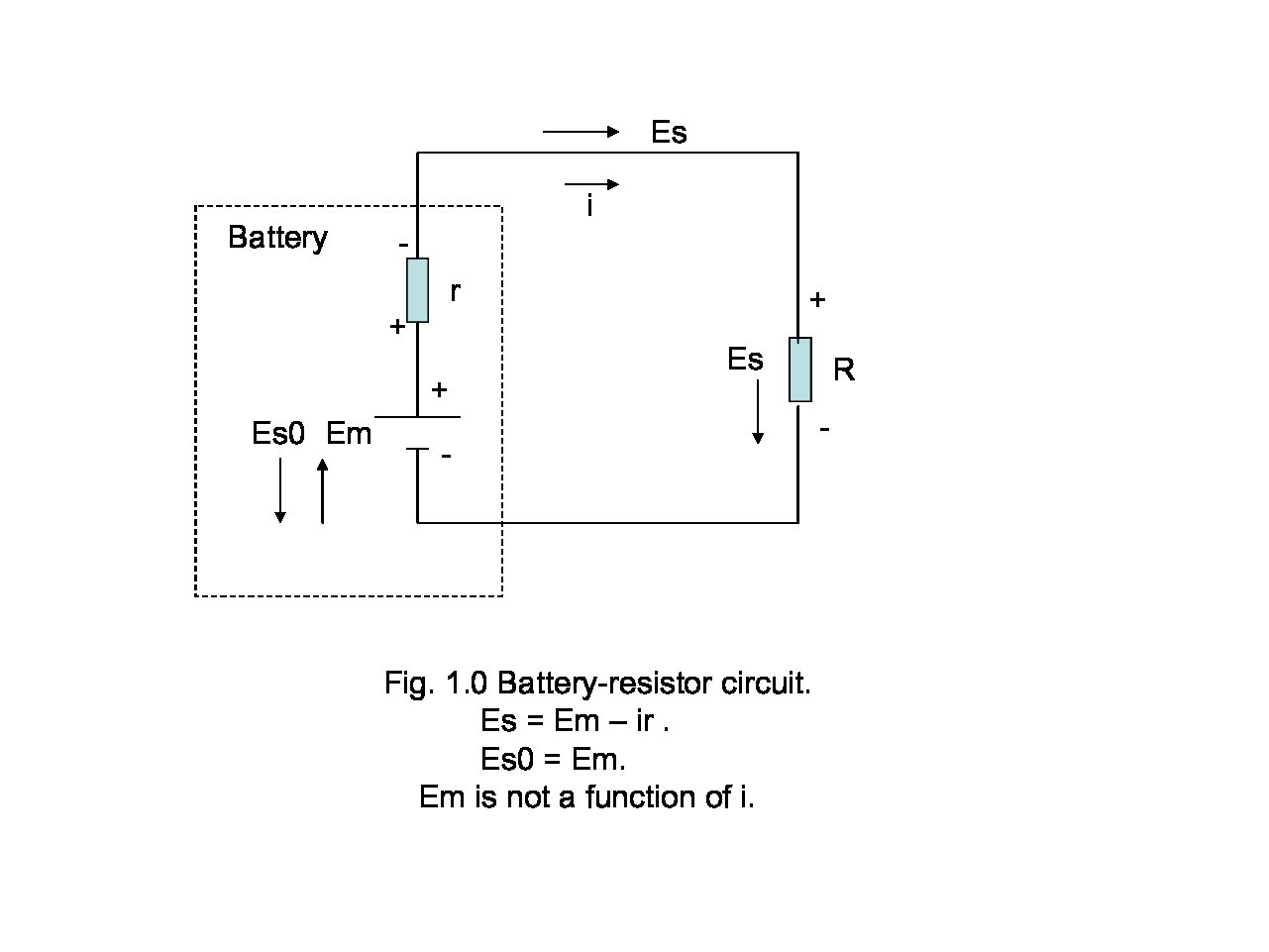
For example, in his battery setup  (Fig. 1) Dr. Lewin assumes a net battery ##E## field opposite to the direction of the ##E## field in the resistor.  Yet the battery has two canceling ##E## fields.  ##E_s## points + to – and ##E_m## points – to +. The line integral of ##E_m## over the length of the battery (- to +)  is the emf of the battery.  A voltmeter senses the line integral of ##E_s## only, otherwise the meter would read 0V DC.  The resistor has an ##E_s## field pointing + to -; the circulation of ##E_s## around the loop is zero as required by Kirchhoff’s voltage law.  The circulation of ##E_m##, and thus ##E##, is ##iR##, ##i## being the current and ##R## the resistor.*

Now to address the main topic here, that of the solenoid, the single-turn loop, and voltmeters positioned as shown in Fig. 2. In what follows, loop resistance is again assumed zero.

Let

##\phi## = magnetic flux inside loop,

loop radius =##a##

loop current = ##i##

total loop induced emf = ## \oint \bf E_m \cdot \bf dl ##

then $$ E_m = \frac {\frac {-d\phi} {dt}} {2\pi a}  $$.

Around the loop with or without the two resistors ##R1## and ##R2## in it, a continuum of ##E_m## field exists throughout the loop, with an ##E_s## field running in the opposite direction.

In the resistors ##E_s## can be very large as ## E_s = iR/d ##  with d the length of each resistor, ##d \ll {2\pi a} ##. All of this follows immediately from the realization that  ##\oint \vec E_s \cdot d \vec l = 0## and the line integral of the two resistors’ ##E_s## fields = ##\mathcal E##, being canceled around the loop by the wire segments’ ##E_s## fields.

Let us next show that a voltmeter as arranged in Fig. 3 and connected at two points a and b along the loop not including a resistor, reads 0V.  That this crucial fact is perhaps paradoxical but entirely logical can be shown as follows:

First, we remind ourselves that ##E_s## and ##E_m## are always equal and opposite in the wire including the shorter section a-b, as well as in the meter leads since a perfect conductor cannot have a net E field.  This is a crucial assumption.

Let

##E_{mw}## = the static field in the meter leads ,

##E_{sw}## = the emf field in the meter leads,

##E_{sv}## = the static field  in the voltmeter,

##E_{mv}## =the emf  field int he voltmeter,

##l_w## = the total meter lead length,

We model the voltmeter as a resistor ##r## of finite physical length ##d##, of arbitrarily high resistance ##r## and  passing correspondingly low current  ##i_v##  with the voltmeter reading ## i_v \cdot r####.

We must then also take cognizance of the fact that, for the voltmeter, ##i_v \cdot r  =  (E_{sv} – E_{mv}) \cdot d ## since ##E_{sv} ## and ##E_{mv} ## oppose. Thus, ##d \cdot E_{sv} = i \cdot r   +   d \cdot E_{mv} ##.

With this in mind, performing the circulation of ##E_s## around the meter circuit,

## +E_{sw} \cdot l_w   +  i_v \cdot r + E_{mv} \cdot d ~  –  ~ E_s\theta a  = 0 ##

The circulation of ##E_m## is also zero since there are no sources of emf within or around that contour:

## E_{mw} \cdot l_w + d \cdot E_{mv}  – E_m \theta a = 0 ##

Combining these last  two, with ##E_{sw} = E_{mw} ## and ##E_s = E_m ## as required,

##i_v\cdot r  = 0,  i_v = 0, VM = 0 ##.

Since VM = 0 for an uninterrupted section of the loop, it follows that the voltage read by a voltmeter probing a length of wire with a resistor ##R## in-between, VM = ##iR## and is not dependent on the length of wire segments surrounding ##R##. This argument includes of course Lewin’s famous A and D points which are located at the top and bottom respectively of the loop as in figs. 2 or 3.

We thus distinguish between VM readings and the so-called “scalar potential” difference which is here ##\int \bf E_s \cdot \bf dl.##  McDonald rightly offers that meter wire coupling is accountable for the difference, which is why he argues for accepting scalar potential differences as the “true” potential difference, not subject to measurement detail.  This is also the explanation offered by Mr. Mabilde. The latter demonstrated an apparently valid way of measuring the scalar potential experimentally with his interior voltage measuring setup, arriving at the correct scalar potential in all cases.  However, his demo is simply a simulation of the Es field profile around the ring, set up by the area of his meter leads..

His statement criticizing Lewin’s “Kirchhoff was wrong” is however spot-on if we understand that the Kirchhoff voltage law applies to voltages in the correct sense of the word, which is the line integral of the Es field only and does not apply to Em fields.

I want to emphasize that the voltmeter readings in Lewin’s setup can be entirely accounted for without splitting the E field into Em and Es.  What I think I contribute is more insight into the observed phenomena.   I believe I have offered a precise explanation for the difference between meter readings and scalar potential differences.  It’s not meter lead dress as suggested by Mr. Mabilde that matters; any meter loop in any orientation will give the same results.  It’s simply ##E_m## and ##E_s## sharing between loops.  As McDonald points out, if you want to avoid the consequences of loop coupling then the meter probes must be connected right at a resistor or the scalar potential difference reading will be wrong since those potentials associated with the loop wiring will not be included..

Lewin did not to my recollection place the VM leads in the middle of the solenoid.  I think we can all accept that the reading would be half-way between -0.1V and + 0.9V, i.e +0.4V.  Reflecting on the numerical mismatch between expected and actual VM readings, we see that the ##R1## meter reading was -0.1V – 0.4V = -0.5V i.e. too negative, while for the ##R2## loop it was +0.9V – 0.4V = +0.5V (too positive).  Now, if we integrate the ##E_s## field over the meter loop with the meter positioned half-way, i.e. directly above, the solenoid, we can sum the line integral of ##E_s## as follows:

## \mathcal E##/2 – ##iR2##  +  VM = 0 or VM  = +0.4V.

Or,  –VM + ##\mathcal E##/2 –##iR1## = 0 also giving VM = +0.4V.  Which agrees with the data.  Suspending the voltmeter with its leads directly above the magnetic source gives the correct voltage.

To summarize, one should be aware of two separate electric fields in the loop and meter lead wiring vs. (essentially) one only in the resistors.  Voltmeters give erroneous voltage readings if the meter circuit forms an alternative path for the Em field, as it does in the Lewin set up and readings.    Coupling effects are predictable and can be shown to be ##E_m## and ##E_s## field sharing between the main loop and the meter loop.  Spurious coupling must be identified and avoided if one wishes to obtain actual scalar potential differences; this may not always be an easy task.

Failure to recognize the two types of ##E## field is bound to lead to confusion or even violation of physics laws in any circuit containing one or more sources of emf, be they batteries, magnetic induction, or any other form of emf generation.

Cf. Stanford Professor Emeritus H. H. Skilling, Fundamentals of Electric Waves, probably out of print but readily available on the Web.

* Footnote added 09/12/2018: since the circulation of the ##E_m## field = ##iR##, by Stokes’s theorem there must also exist net curl within the contour.  If we set up  x-y-z coordinates in fig. 1 with the contour in the x-y plane, x axis at the neg. terminal of the battery, y axis coincident with just inside the battery on its right-hand side, L = battery length neg. to pos. terminal, then ## iR = \oint \vec E_m \cdot d \vec l = \iint_S (\nabla \times \vec E_m) \cdot d \vec A ##.
A curl that satisfies this equation is ## \nabla\times\vec E_m = \frac {\partial E_m} {\partial x}~ {\mathbf k}  = E_m \delta(x)~ {\mathbf k} ##.  Then ## \iint_S (\nabla\times\vec E_m) \cdot d \vec A = \int_{0-}^{L+} E_mL~ \delta(x) \, dx = E_mL ##  as expected.

References:

  1. https://www.youtube.com/watch?v=FUUMCT7FjaI
  2. https://www.physicsforums.com/threads/faradays-law-circular-loop-with-a-triangle.926206/page-4
  3. Attachment: K. McDonald: “Lewin’s Circuit Paradox”
  4. Attachment: K. McDonald, “What Does a Voltmeter Measure?”

 

 

You might also like
electromagnetic computations dualityA Numerical Electromagnetic Solver Using Duality
model magnetHow to Model a Magnet Falling Through a Conducting Pipe
model magnetHow to Model a Magnet Falling Through a Solenoid
electrical wireMisconceiving Mutual Inductance Coefficients
How to Recognize Split Electric Fields
Milli-OhmThe Poor Man’s Milli-Ohm Meter
65 replies
Newer Comments »
  1. rude man says:
    anorlunda

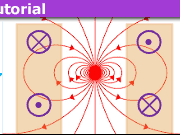
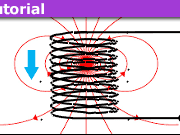
    At minutes 30-35 in the video, he says that Kirchoff's Laws only apply when the external magnetix flux is zero. And since the flux is nonzero in his experiment (assumption #1) you can't use Kirchoff's Laws or circuit analysis to describe that experiment. Well duh. :rolleyes:

    Bottom line, you can't say that KVL and KCL apply always.

    By the way, be careful when you say you don't agree with those assumptions. They are repeated in many standard textbooks. Peer reviewed journals and standard textbooks are the bible here on PF.Voltage is the line integral of the electrostatic field. And the circulation of that field is zero.
    I suggest perusal of the two papers by Princeton's K. McDonald I cited in my Insight article on this subject.
    And if I may counter with my own "standard textbook": Fundamentals of Electric Waves by Stanford's H H Skilling. Let me know if you need chapter & pages.

  2. anorlunda says:
    rude man

    The Lewin setup includes time rate of change of magnetic flux outside a conductor being non-zero if I'm interpreting your statement per your intention, yet there Kirchhoff's laws certainly hold.At minutes 30-35 in the video, he says that Kirchoff's Laws only apply when the external magnetix flux is zero. And since the flux is nonzero in his experiment (assumption #1) you can't use Kirchoff's Laws or circuit analysis to describe that experiment. Well duh. :rolleyes:

    Bottom line, you can't say that KVL and KCL apply always.

    By the way, be careful when you say you don't agree with those assumptions. They are repeated in many standard textbooks. Peer reviewed journals and standard textbooks are the bible here on PF.

  3. rude man says:

    I think I disagree with your #1. The Lewin setup includes time rate of change of magnetic flux outside a conductor being non-zero if I'm interpreting your statement per your intention, yet there Kirchhoff's laws certainly hold.

    I agree with the rest. Quasi-stationariness must be assumed, ortherwise lumped-circuit anaylis laws have to be superseded by Maxwell's equations. A radiating circuit is one example, as is a distributed circuit.

    But that is not the discussion here.

    Reference https://www.physicsforums.com/insights/circuit-analysis-assumptions/

  5. rude man says:
    arydberg

    I did this experiment and it shows what Dr. Lewin stated. I took a 500 foot roll of wire used for dog fencing. ( it measured 5 ohms). Unspooled it, Cut it in half and rewound 250 feet back on the spool. I brought out a pigtail and connected it to a 1meg resistor. The other end of the resistor went to the 2nd 250 foot wire. I then wound the 2nd piece on the spool in the same direction. and connected a 100 K resistor to the beginning and end of the coil.

    I put 2 strong magnets in the center of the coil and snatched them away and got a pulse on my Oscilloscope. The pulse on the 1 meg resistor was 10 times that on the 100 K resistor. I have 2 pictures and hopefully included them.Dr. Lewin's data was never in contention. That was not the issue. It's his explanations that were wrong, in particular the statement that "Kirchhoff was wrong". Kirchhoff's laws hold in all cases. They refer to voltage drops, which is not necessarily the same as measurements using voltmeters.

    A voltmeters always correctly measures the voltages it "sees" but this voltage can be artificially induced by the voltmeter and its leads and is thus not the voltage in the absence of the voltmeter and its leads. For example, in Lewin's setup there is a voltage between any two points along a wire not including a resistor, yet the voltmeter reads zero.

    The sum of voltages along any closed path is always zero irrespective of the nature of the emf generating them.

  6. arydberg says:

    I did this experiment and it shows what Dr. Lewin stated. I took a 500 foot roll of wire used for dog fencing. ( it measured 5 ohms). Unspooled it, Cut it in half and rewound 250 feet back on the spool. I brought out a pigtail and connected it to a 1meg resistor. The other end of the resistor went to the 2nd 250 foot wire. I then wound the 2nd piece on the spool in the same direction. and connected a 100 K resistor to the beginning and end of the coil.

    I put 2 strong magnets in the center of the coil and snatched them away and got a pulse on my Oscilloscope. The pulse on the 1 meg resistor was 10 times that on the 100 K resistor. I have 2 pictures and hopefully included them.

  7. rude man says:
    Charles Link

    You are referring to the electric field "inside the conductor". The "inside the conductor" part was not clearly stated. (Outside the conductor, the electric fields do not cancel).No I'm not. I'm referring to the net axial E field, comprising equal and opposite ##E_m## and ##E_s## fields. Shouldn't be much argument as to what "axial" means IMO.

    The E field in the wire is zero if the wire has zero resistance and is entirely ##E_m## if the resistance is finite.

  8. rude man says:
    vanhees71

    Why is ##-vec{nabla} phi## necessarily 0? In my opinion the problem description is to incomplete to make a statement about the source part of ##vec{E}## simply because the sources of the em. field are not specified.Referring back to post 22, I was referring to the circular E fields within the solenoid, which are in air, for which obviously there can be no static component, ergo no potential gradient.

    If you're thinking of the axial E fields along the solenoid – there are two E fields, one is emf-generated and one is static. They cancel each other so there is no net axial E field in the solenoid.

  9. vanhees71 says:

    That's not precisely the one I meant, but it seems to be good too (I'll watch it completely later this weekend). What I had in mind was a collection of an entire introductory experimental physics course with a lot of experiments done by Lewin in the lectures. Originally it was at MIT, but it's also still on Youtube, as your link shows. I think, this one is the movie I had in mind:

  10. vanhees71 says:

    BTW: This also applies to Walter Lewin's paradox either, and this is a kind of paradox which can be even easier avoided than the twin paradox, because it's due to sloppy language, i.e., because some people call an EMF a voltage, although a voltage is a potential difference. As Faraday's Law, ##vec{nabla} times vec{E}=-partial_t vec{B}## explicitly states (again math is your friend) this is precisely a situation where the fundamental concept is that ##vec{E}## in this case has no potential and thus that line integrals between two points are path dependent, and that's also very nicely explained by Lewin in his famous lectures (I hope they are still available on Youtube although he stupidly ruined his reputation by stupid emails to students).

  11. vanhees71 says:

    I see what you mean now. You want to calculate ##vec{E}## in the hand-waving way how sometimes the electric field of a statically charged sphere or infinitely long cylinder is calculated by making use of the integral laws. Well, this is only possible for very very symmetric situations and a solid portion of physics intuition not to make mistakes with hand-waving arguments. In general, this is something for magician genisuses rather than us usual mortals, who better use math. Of course, for sufficiently symmetrical problems the math becomes usually very simple, as this example shows.

    Another observation is that almost everything which is dubbed "a paradox" in physics is simply due to the fact that some people rather obscure the didactics by trying to avoid math, making the understanding of the problem at least difficult if not impossible and then leading to apparent contradictions. The prime example is the so-called twin paradox, usually presented in the first few lectures about special relativity, instead of simply stating that an ideal clock always shows its proper time. It's anyway wrong to present special relativity as a collection of apparent paradoxes rather than stressing that it resolves the paradoxes of Newtonian physics, but that's another topic.

  12. vanhees71 says:
    rude man

    @Charles, that is what I responding to. I think you're right, you can't assume symmetry of the E field around the off-axis solenoid; all you can assume is the circulation of E = -d(phi)/dt. And as I said it's like trying to apply Ampere's law to a finite wire: the circulation of H = I always, but that H is not uniform around any circular path. I remember getting faked out (temorarily only of course ha ha) just this way.

    As far as vanHees' post is concerned, there is no E[SUB]s[/SUB] field anywhere around your path so the gradient term -∇Φ is zero so that does not seem to offer any further enlightenment. I can only think that you have to solve ∇xE = ∇xE[SUB]m[/SUB] at every point along your chosen path which is probably prohibitively difficult.

    In your case, going with polar coordinates, ∇xE = [∂E[SUB]φ[/SUB]/∂r+ E[SUB]φ[/SUB]/r – (1/r) ∂E[SUB]r[/SUB]/∂φ] k = -∂B/∂t. With the third term on the LHS non-zero plus the boundary values that would be more than the feeble math knowledge of a dumb EE like myself could handle!

    EDIT :even if your path doesn't include any B so that ∂B/∂t = 0 the problem is probably not much easier!Why is ##-vec{nabla} phi## necessarily 0? In my opinion the problem description is to incomplete to make a statement about the source part of ##vec{E}## simply because the sources of the em. field are not specified.

    Charles Link

    Yes, thank you, I have completely solved this one. I do believe I have seen textbooks that present the problem of a uniform ## frac{dB}{dt}=beta ## into the plane of the paper and ask you to compute ## E ## around an arbitrary circle. ## \ ## The long solenoid with current ## I(t)=alpha t ## does have uniform ## frac{dB}{dt}=beta ##, (in the ## hat{z} ## direction), throughout its interior. The surprising thing is it is incorrect to pick an arbitrary circle to compute ## E ## and assume uniformity of ## E ##. The circle must be centered on the axis of the solenoid, or ## E ## is not constant, (in the ## hat{a}_{phi}## direction), around the circle. The computation of the EMF ## mathcal{E}=oint E cdot dl ## works, but a uniform ## frac{dB}{dt} ##, surprisingly, doesn't have sufficient symmetry to compute ## E ## from the uniform ## frac{dB}{dt} ## inside the circle. ## \ ## The ## E ## gets computed everywhere by drawing circles of varying radii, that are all centered on the axis of the solenoid. For these circles, ## mathcal{E}=oint E cdot dl=2 pi , r , E(r) =-pi r^2 frac{dB}{dt}=-beta pi r^2 ##.Let's see again. I still don't get this obvious contraction of fundamental math, in this case Stokes's integral theorem.

    Let's do it once more: As usual, the local treatment is the most simple approach. You have given the (approximate) magnetic field
    $$vec{B}=beta t vec{e}_3.$$
    Then we have
    $$vec{nabla} times vec{E}=-partial_t vec{B}=-beta vec{e}_3.$$
    The solution of this is
    $$vec{E}=-beta vec{r} times vec{e}_3-vec{nabla} Phi,$$
    where ##Phi## is undetermined since the sources are not given.

    For the EMF this is of course unimportant since any closed-loop integral over a gradient in a simply connected region (which is the case for the interior of the solenoid you are discussing) gives zero. Thus we have
    $$int_{partial A} mathrm{d} vec{r} cdot vec{E}=int_{A} mathrm{d}^2 vec{f} cdot (vec{nabla} times vec{E}) = -int_{A} mathrm{d}^2 vec{f} cdot beta vec{e}_3.$$
    For any (!!!) circle of radius ##R## parallel to the ##x_1 x_2## plane completely in the interior of your solenoid gives thus ##-pi beta R^2##. What's, in your opinion, wrong with this simple argument?

  13. vanhees71 says:
    rude man

    @Charles, that is what I responding to. I think you're right, you can't assume symmetry of the E field around the off-axis solenoid; all you can assume is the circulation of E = -d(phi)/dt. And as I said it's like trying to apply Ampere's law to a finite wire: the circulation of H = I always, but that H is not uniform around any circular path. I remember getting faked out (temorarily only of course ha ha) just this way.

    As far as vanHees' post is concerned, there is no E[SUB]s[/SUB] field anywhere around your path so the gradient term -∇Φ is zero so that does not seem to offer any further enlightenment. I can only think that you have to solve ∇xE = ∇xE[SUB]m[/SUB] at every point along your chosen path which is probably prohibitively difficult.

    In your case, going with polar coordinates, ∇xE = [∂E[SUB]φ[/SUB]/∂r+ E[SUB]φ[/SUB]/r – (1/r) ∂E[SUB]r[/SUB]/∂φ] k = -∂B/∂t. With the third term on the LHS non-zero plus the boundary values that would be more than the feeble math knowledge of a dumb EE like myself could handle!

    EDIT :even if your path doesn't include any B so that ∂B/∂t = 0 the problem is probably not much easier!Why is ##-vec{nabla} phi## necessarily 0? In my opinion the problem description is to incomplete to make a statement about the source part of ##vec{E}## simply because the sources of the em. field are not specified.

  14. rude man says:
    Charles Link

    I think we are very fortunate in this case to have a long solenoid, with current ## I(t)=alpha , t ##, that is able to generate exactly what we need in terms of a uniform B with a ## frac{dB}{dt}=## constant, into the paper, so that we have a practical apparatus to make such a magnetic field. Otherwise it becomes a case where the EMF can be computed from Faraday's law, but not the electric field ## E ##. ## \ ## I do think it is likely the solenoidal geometry proved very important for Faraday and others in coming up with the understanding of magnetism that we presently have. ## \ ## It is not immediately obvious from Biot-Savart or Ampere's law, but detailed calculations do show that ## B ## is completely uniform inside a long solenoid with ## B=mu_o , n , I , hat{z} ##.If the contour and B field are circular and concentric, you can compute the E field everywhere along the contour, whether the contour is inside or outside the B field (solenoid).

    If the path is irregular and the B field is circular you can use your variable-radius technique to determine E everywhere along the contour.

    Agreed?

  15. rude man says:

    I agree with what you say. I still don't see why it's surprising. Interesting but not surprising. I don't think I would ever have assumed otherwise even before your posts but who knows. I cite again the analogy with Ampere's law (mis)applied to a finite-length wire. Circulation of H: check. Detailed knowledge of H: X. In the ampere case the finite length of wire must produce a B field from the returning wiring which distorts the H field. And so with an external B field generating separate E fields which will distort the symmetry of the local E field. Quite complementary, looks like.

    Given an arbitrary configuration of the B field covering and surrounding your closed path would mean solving for E[SUB]r[/SUB] and E[SUB]φ[/SUB] in Maxwell's equation which would be hard or impossible in closed form. In any case I fail to see what's surprising about the non-uniform E field.

  16. rude man says:

    Anyway, my equation in post 26 must apply and is valid irrespective of surroundings. The thing is you know dB/dt everywhere along the closed circular path but you'll never find the boundary values on E[SUB]φ[/SUB] and E[SUB]r[/SUB]. Or so I think.

  18. rude man says:

    Great idea, using varying radii about the B field center to find the E field anywhere around any contour! But that still assumes a circular B field, doesn't it? Of course, that's what you get with your long solenoid. Anyhow, you thereby answered your question and why does the lack of symmetry surprise you? Varying radii will give varying E field magnitudes and directions as you go around your contour, right? Nothing symmetrical there.

  19. rude man says:
    Charles Link

    @vanhees71 @rude man Please see the "Edit" at the bottom of post 22=that is the solution to this problem that puzzled me.@Charles, that is what I responding to. I think you're right, you can't assume symmetry of the E field around the off-axis solenoid; all you can assume is the circulation of E = -d(phi)/dt. And as I said it's like trying to apply Ampere's law to a finite wire: the circulation of H = I always, but that H is not uniform around any circular path. I remember getting faked out (temorarily only of course ha ha) just this way.

    As far as vanHees' post is concerned, there is no E[SUB]s[/SUB] field anywhere around your path so the gradient term -∇Φ is zero so that does not seem to offer any further enlightenment. I can only think that you have to solve ∇xE = ∇xE[SUB]m[/SUB] at every point along your chosen path which is probably prohibitively difficult.

    In your case, going with polar coordinates, ∇xE = [∂E[SUB]φ[/SUB]/∂r+ E[SUB]φ[/SUB]/r – (1/r) ∂E[SUB]r[/SUB]/∂φ] k = -∂B/∂t. With the third term on the LHS non-zero plus the boundary values that would be more than the feeble math knowledge of a dumb EE like myself could handle!

    EDIT :even if your path doesn't include any B so that ∂B/∂t = 0 the problem is probably not much easier!

  20. rude man says:

    Charles Link@vanhees_71 What happens if we choose a circle that does not have the origin at the center? Does that mean the symmetry of ## E ## around the circle is no longer applicable?You mean with a circular B field centerd at the origin I assume. I would think the symmetry is then shot. The only guarantee is in the form of the circulation of E, not symmetry of E.. LIke trying to apply Ampere's law to a finite-length wire.

    In ##∇xE = – ∂B/∂t ## with ## E = E[SUB][/SUB][SUB]m[/SUB] + E[SUB][/SUB][SUB]s[/SUB] ## the ## E[SUB]s[/SUB] ## of course does not contribute to the curl. ## E[SUB]s[/SUB] ## is the ## -∇φ ## of post 19 ## .
    ##
    BTW about your two circles touching – I offhand would say there is no effect on either ring after contact is made. Each ring has its E[SUB]m[/SUB] field in the same counterclockwise direction. At the point of contact the fields cancel but I see no issue with this. E fields, both emf and static, can exist alongside, linearly augmenting or canceling each other to some extent. 'Bout all I have to say I guess.

  21. vanhees71 says:

    I still don't get your problem. Obviously your electric field is fulfilling Faraday's Law (maybe I misunderstand your undefined notation again since you don't tell what ##vec{j}## is; I don't see any current density explicitly mentioned in the problem).

    As I argued, for your setup the electric field reads
    $$vec{E}=-1/2 vec{r} times vec{beta}-vec{nabla} phi = -beta/2(x_2,-x_1,0).$$
    If I understand you right, we suppose ##vec{beta}=beta vec{e}_3## and
    Circle 1 is parametrized by
    $$vec{r}(varphi)=(R cos varphi,R sin varphi,0).$$
    The gradient part vanishes when integrated over the closed circle (since there shouldn't be any further "potential vortex like" singularities in this part). Thus we have
    $$int_{C_1} mathrm{d} vec{r} cdot vec{E}=int_{0}^{2 pi} mathrm{d} varphi frac{mathrm{d} vec{r}}{mathrm{d} varphi} cdot vec{E} = pi R^2 beta.$$
    For Circle 2 you have
    $$vec{r}=(R cos varphi,R sin varphi,0)+(2R,0,0).$$
    Since the electric field is assumed to be homogeneous in the entire range of consideration the evaluation of the EMF is literally the same as for circle 1 and thus of course yields the same result. You can of course also integrate the magnetic field over the corresponding circular disk, showing that everything is consistent also with the integral form of Faraday's Law as it must be thanks to Stokes's integral theorem.

  22. vanhees71 says:

    There's nothing wrong with your calculation. If the field is homogeneous in the region you get of course always ##-beta A##, provided you integrate over a plane area perpendicular to ##vec{beta}##. So I don't understand your statement that you get something else for another circle. As long as you are in the region where the fields are homogeneous, there's no difference where you put the origin of the circle (it only must be entirely in the region where the fields are homogeneous).

    Of course, this is just math. It's hard to imagine how to produce such fields in reality.

  23. vanhees71 says:

    Of course only knowing ##vec{nabla} times vec{E}## is not sufficient to calculate the field. It determines the field only up to a gradient. I'm not sure, whether this is a sound example, because it's not clear to me whether your setup fulfills all of Maxwell's equations. Only solutions that fulfill all Maxwell equations are consistent in describing a situation.

    I also don't understand the very beginning of your argument.

    Suppose (without thinking much about the physicality of the assumptions) there's a region, where
    $$vec{B}=vec{beta} t$$
    with ##vec{beta}=text{const}##. You get
    $$vec{nabla} times vec{E}=-vec{beta}=text{const}.$$
    This implies
    $$vec{E}=frac{1}{2} vec{r} times vec{beta} -vec{nabla} phi$$
    for an arbitrary scalar field ##phi##.

    Up to this gradient the electric field is unique, and thus also the EMF is uniquely defined for any closed loop, giving by construction the ##-dot{Phi}## with the flux according to this loop. Maybe I simply misunderstand your description. Perhaps you can give your concrete calculation to look into the issue further.

  24. rude man says:

    Oops, missing post? Somebody asked,
    Hi
    In figure 2 inside the resistors, do the Em and Es fields add and help rather than oppose like they do in the connecting wire?

    The answer is yes, they are both clockwise in the figure. E[SUB]m[/SUB] is always clockwise but in a resistor the E[SUB]s[/SUB] field points + to – so they add.
    Line-integrated E[SUB]m[/SUB] will be small, especially if the resistor is short, but line -inegrated (E[SUB]s[/SUB] + E[SUB]m[/SUB]) = iR so you can see that E[SUB]s[/SUB] is the dominant field in R.

  25. rude man says:

    I have made some emendations to the original blog. Aside from a few typo corrections etc. I have simplified the math and removed the assumption of finite resistor and meter physical dimensions which were implicit in the original version.

  26. rude man says:
    Charles Link

    @rude man The EMF from a battery seems to be of a slightly different nature than the EMF of Faraday's law. I was trying to come up with an analogy that might describe the type of mechanism involved where the chemical reactions create a potential difference resulting in an electrostatic field: One perhaps related mechanism would be a spring system that pushes apart positive and negative charges: e.g. You could have two capacitor plates, initially at ## d=0 ## with one having positive charge and the other negative charge. The spring system could supply energy to push them apart and create a voltage. In this case, electrostatic fields would be generated having ## oint E_s cdot ds =0 ##. The force from the spring is quite localized and is essentially in the form of an EMF. The external loop could be completed between the two plates with a large resistor that could essentially be the resistance of a voltmeter. ## \ ## Once again, the equations are quite similar, and agree with the concept your Insights article promotes, that the voltmeter actually measures the integral of the electrostatic field ## E_s ## external to the battery. Sounds good.
    Somewhere I mentioned another analogy I liked, given by Prof. Shankar of Yale. He likened the process to a ski lift; you need force (Em) to overcome gravity (Es) to get from the bottom to the top, then you ski down the slope (current thru the resistor) but you bump into trees along the way (heat dissipation) so when you get to the bottom you have zero k.e. Then you repeat the process. He mentions this analogy again later when he discusses induction so I still think the two are very much the same thing except as you point out a real battery has internal resistance in which case the Es field has to be reduced from |Em| to allow for the excess Em to push the charges thru the internal R.

    I highly recommend Prof. Shankar's youtube lectures. I have picked up a lot from them and am still absorbing.

    Thanks for continuing the discussion!

  27. rude man says:
    Charles Link

    The inductor is a conductor (It is made of conducting wire, with typically very ideal conduction). The current density at any location is given by ## vec{J}=sigma vec{E} ##, where the conductivity ## sigma ## is quite large and essentially nearly infinite. When a conductor experiences an electric field and is part of a loop with a resistor, the resistor will limit the current density and make it quite finite. In order to have the same finite current everywhere in the loop, there will be a redistribution of electrical charges in the conductor, and the redistribution is such as to create a static ## vec{E}_s ## that will make the current and current density finite. In order to do this, this implies ##vec{E}=vec{E}_{total} approx 0=vec{E}_s+vec{E}_{induced} ## in the inductor. ## \ ## In the case of a chemical battery, there normally is an internal resistance, so the full voltage is only measured in a nearly open circuit configuration, with a voltmeter with a large resistance. In the case of a chemical battery, (which because of the internal resistance has a very finite conductivity ## sigma ##), with a smaller load resistor in the loop, ## vec{E}=vec{E}_{total} ## could certainly be non-zero. ## \ ## Perhaps the thing that each of these cases has in common is that the Kirchhoff Voltage Laws (KVL) always apply. To get to the reason behind why KVL works, it does help to treat separately the electric fields ## E_s ## and ## E_{induced} ##, as you @rude man have done in your Insights article. Once again, very good reading. :smile:Thanks CL.

  28. Ronald Hamilton says:

    If the covenant says Abraham I will make your descendants as many as the stars, and latest research estimates 2 trillion galaxies with each having about 10,000,000 billion stars which is give or take an inch about 10 the 27th, and the earth's carrying capacity is estimated at about 10 billion how much energy and time is required to complete such a task? What speed must we achieve for this?

Newer Comments »

Leave a Reply

Want to join the discussion?
Feel free to contribute!

Leave a Reply