An Introduction to Theorema Primum
Table of Contents
Introduction
Whilst no doubt most frequenters of “Physics Forums” will be familiar with Nicolaus Copernicus as the scientist who advanced the (at the time) radical thesis that the earth revolved around the sun rather than vice versa, a perhaps less well-known aspect of his work is the “nuts and bolts” geometry underlying his ground-breaking treatise: “De Revolutionibus Orbium Coelestium” (On the Revolutions of the Heavenly Spheres). In this article (and perhaps others to follow), we analyse “Copernican geometry” in light of its stated intent:
Because the proofs which we shall use in almost the entire work deal with straight lines and arcs, with plane and spherical triangles, and because Euclid’s Elements, although they clear up much of this, do not have what is here most required, namely how to find the sides from the angles and the angles from the sides, since the angle does not measure the subtending straight line – just as the line does not measure the angle – but the arc does, there has accordingly been found a method whereby the lines subtending the arc may become known. By means of these lines or chords, it is possible to determine the arc corresponding to the angle; and conversely by means of the arc to determine the straight line or chord which subtends the angle … But we will unfold this in six theorems and a problem – following Ptolemy fairly closely.
Theorema Primum (First Theorem)
The diameter of a circle being given (ie 200000 parts), the side of the triangle, tetragon, pentagon, hexagon and decagon, which the same circle circumscribes, are also given.
Half the diameter (or the radius), is equal to the side of the hexagon (Euclid IV,15); the square on the side of the triangle is three times the square on the side of the hexagon (Euclid XIII,12); and the square on the side of the tetragon is twice the square on the side of the hexagon (Euclid IV,9 and I,47). Therefore the side of the hexagon is given in length as 100000 parts, that of the tetragon as 141422 parts and that of the triangle as 173205 parts.
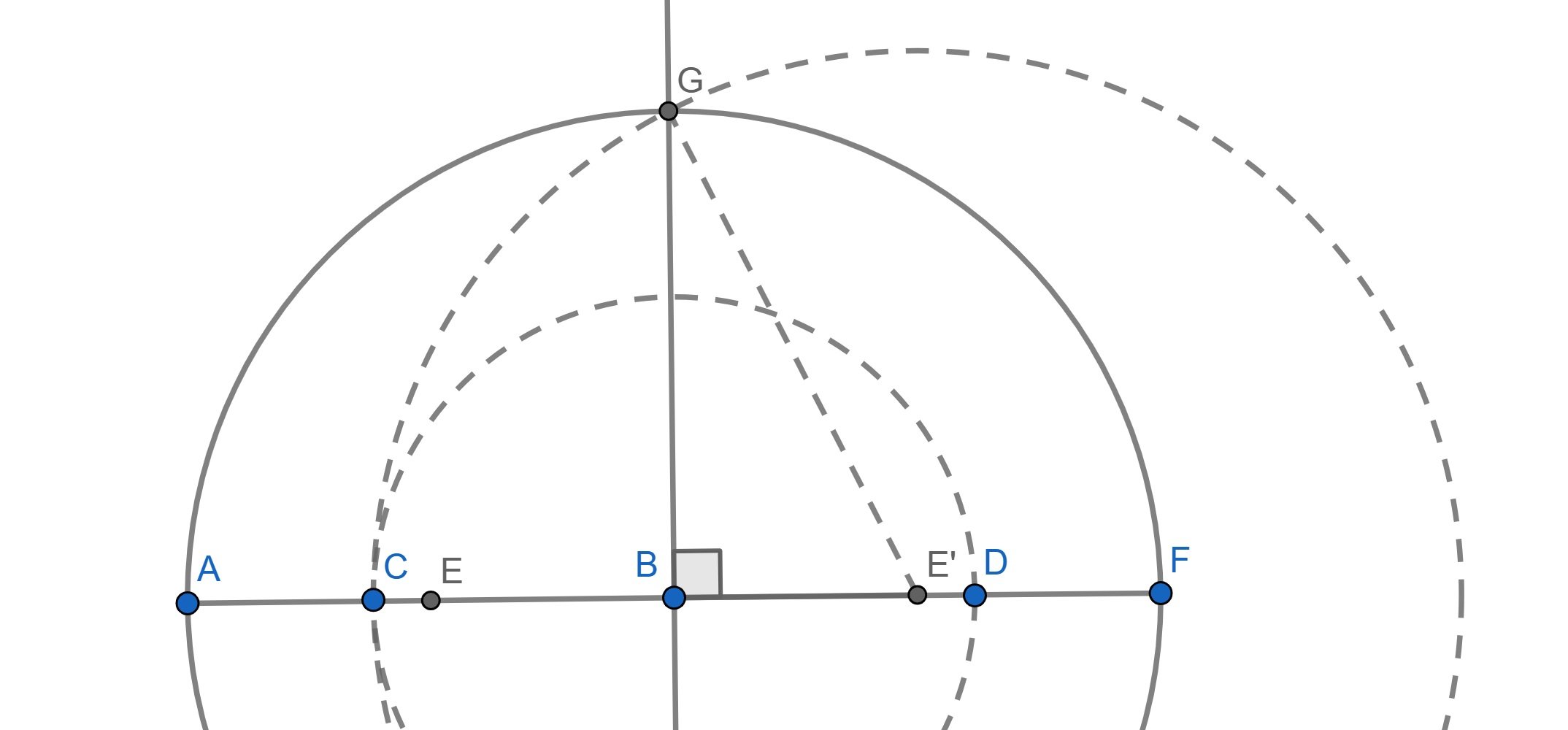
Now let AB be the side of the hexagon (ie the circle radius) and by Euclid II, 11 or VI, 30, let it be cut in mean and extreme ratio at point C; and let CB be the greater segment to which its equal BD is added. Therefore the whole ABD will have been cut in mean and extreme ratio and the lesser segment BD will be the side of the decagon inscribed in the circle and AB will be the side of the inscribed hexagon as is made clear by Euclid XIII: 5 and 9.
But BD will be given in this way: let AB be bisected at E, and it will be clear from Euclid XIII:3 that $${EBD}^2=5\times{EB}^2.$$ But $$EB=50000,$$ whence $$5\times{EB}^2$$ is given. Hence $$EBD=111803.$$ And $$BD=EBD-BD=111803-50000=61803$$ which is the side of the decagon sought.
Moreover, the side of the pentagon, the square on which is equal to the sum of the squares on the side of the hexagon and on the side of the decagon (Elements; XIII:10), is given as 117557 parts.
Therefore the diameter of the circle being given, the sides of the triangle, tetragon, pentagon, hexagon and decagon, which may be inscribed in the same circle, have been given – as was to be shown.
Porism (aka Pythagoras’ Theorem)
Furthermore, it is clear that when the chord subtending an arc has been given, that chord too can be found which subtends the rest of the semicircle.
Since the angle in a semicircle is right, and in right triangles, the square on the chord subtending the right angle (ie the square on the diameter) is equal to the sum of the squares on the sides comprehending the right angle; therefore – since the side of the decagon which comprehends ##36^{\circ}## of the circumference has been shown to have 61803 parts whereof the diameter has 200000 parts – the chord which subtends the remaining ##144^{\circ}## of the semicircle has 190211 parts.
And in the case of the side of the pentagon, which is equal to 117557 parts of the diameter and subtends an arc of ##72^{\circ}##, a straight line of 161803 parts is given, and it subtends the remaining ##108^{\circ}## of the circle.
Analysis
Constructions to inscribe an equilateral triangle, ‘tetragon’ (square) and regular hexagon in a circle along with calculation of their sides (in terms of circle diameter) should be obvious enough. These lead to the well-known trigonometric ratios of “special angles” which every introductory course in trigonometry will include. Nevertheless, we will include simple construction diagrams for these figures for the sake of completeness. We will also demonstrate how the calculated sides of regular ‘n-gons’ translate into the sine values of the associated angles or (correspondingly) into the values of the “half chords” presented in the Copernican table – the endpoint of the “six theorems and a problem” mentioned in the introduction above.
Inscribed Triangle and Hexagon
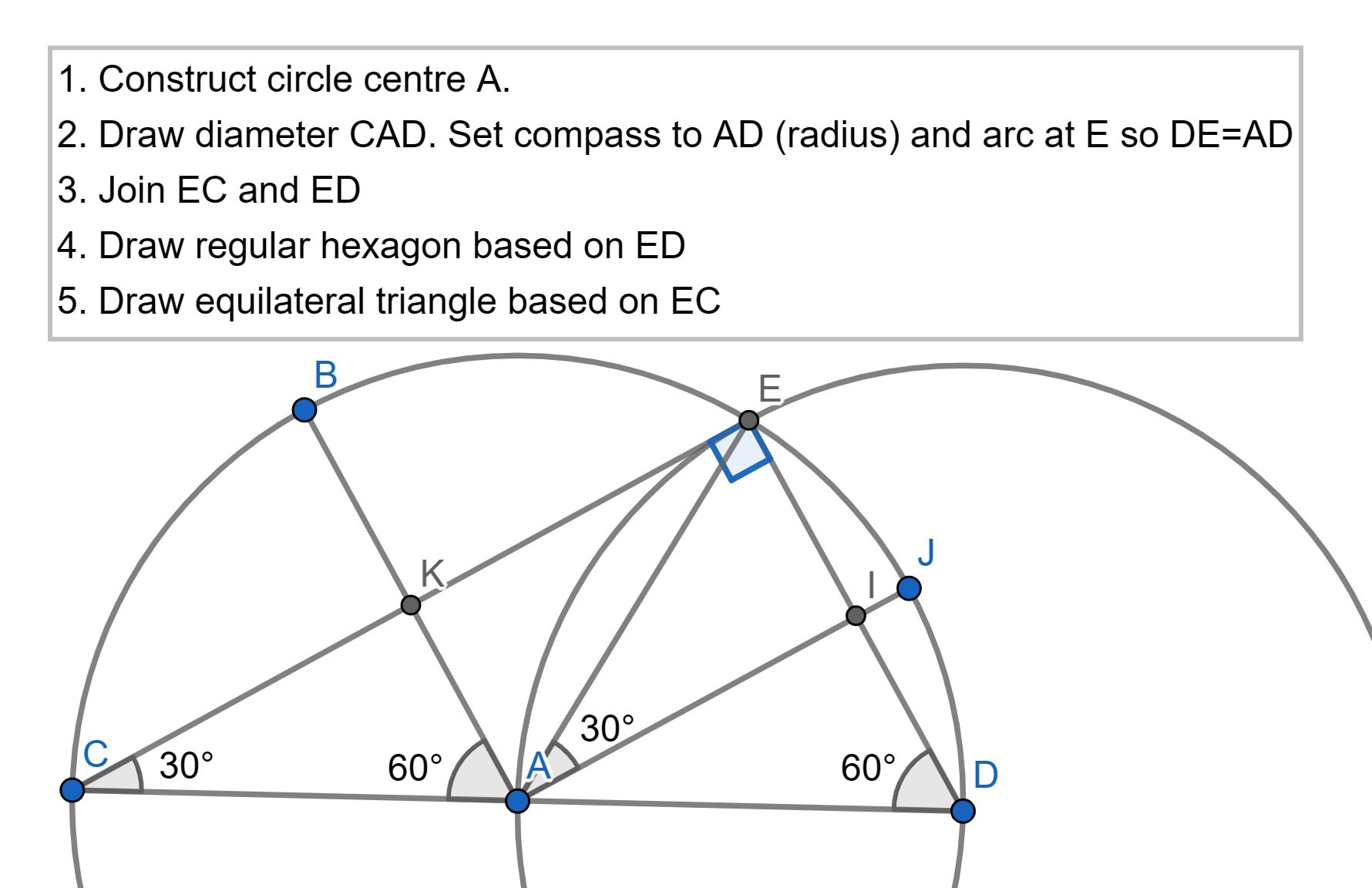
Here chord CE is one side of an inscribed equilateral triangle and DE of an inscribed regular hexagon. If the circle diameter is given as 200000 units, it should be clear that chord DE is of length 100000 units and (by Pythagoras theorem) chord EC will be 173205 units. Note that for his table entries, Copernicus takes “half the chord subtended by twice the arc.” So here for example CB is a ##60^{\circ}## arc and “twice the arc” is CBE which is subtended by chord EC. So the table entry against ##60^{\circ}## is half of chord EC or 86603 units. Similarly, EJ is a ##30^{\circ}## arc and “twice the arc” is EJD. The table entry against ##30^{\circ}## is half of chord ED which is 50000 units. These entries correspond very simply to ##100000 \sin(x^{\circ})##.
Inscribed “Tetragon” (square)
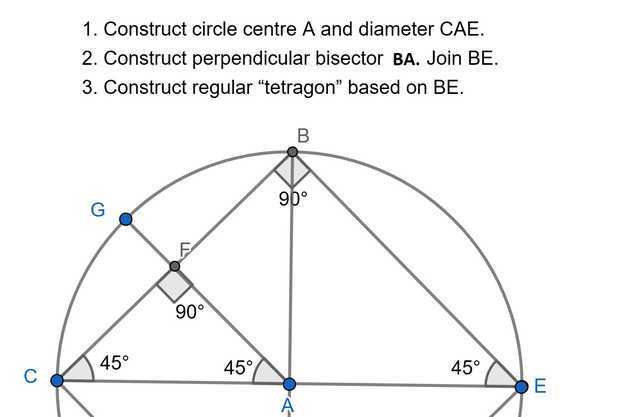
With radius AE 100000 units, side BC will be 141421 units. CG is a ##45^{\circ}## arc and so the corresponding entry in the table will be half of chord BC = 70711 units.
Inscribed Decagon and Pentagon
Less well-known are the “special angles” corresponding to the various chords associated with the inscribed decagon and pentagon. Here we need to explain the ancient geometric technique of dividing a line into “extreme and mean”: that is of dividing a line such that the ratio of the greater part to the lesser is equal to the ratio of the whole to the greater.
Let line AB be divided according to the ratio k:1-k in which k<1 and k>1-k. Hence according to the above ‘prescription’: $$\frac{k}{1-k}=\frac{1}{k}\implies k^2+k-1=0\implies (k+½)^2=\frac{5}{4} \implies k=\frac{-1 \pm \sqrt{5}}{2}.$$ We will use the letter ##\phi## for the solution ##k=\frac{\sqrt{5}-1}{2}\approx 0.618034## – this number has the interesting property that if we add 1 to it , we obtain its reciprocal ie: ##1+\phi=\phi^{-1}=\Phi \approx 1.618034.## Readers may be familiar with ##\Phi## as the well-known ‘golden-ratio’ but in this article we will express various chord lengths (associated with the pentagon and decagon) in terms of ##\phi## rather than ##\Phi.## We should now be able to make more sense of the diagram presented earlier:

Radius BG is constructed perpendicular to AB. E’ is the midpoint of radius BF and is joined to point G. A construction circle with centre E’ and radius GE’ is drawn so that GE’=CE’ will both be ##\frac{\sqrt{5}AB}{2}##. Therefore: $$CB=CE’-BE’=\frac{\sqrt{5}AB}{2}-\frac{AB}{2}=\frac{(\sqrt{5}-1)AB}{2}=\phi AB$$. Copernicus further notes that if the equal of CB (ie BD) is added to AB, the whole ABD will be divided in “extreme and mean” with the greater portion being the radius AB (side of the inscribed hexagon) and the lesser being BD=BC (side of inscribed decagon). $$\frac{AB}{BD}=\frac{AD}{AB}\implies{AB}^2=BD.AD={AB}^2.\phi(\phi+1)={AB}^2$$ since, as we have earlier observed, ##\phi+1=\phi^{-1}##. We have stated but not yet proved that BD and BC (##\phi AB##) will both be equal to the side of the inscribed decagon. The proof comes from Euclid XIII, 9 which states:
If the side of the hexagon and that of the decagon inscribed in the same circle are added together, then the whole straight line has been cut in extreme and mean ratio, and its greater segment is the side of the hexagon.
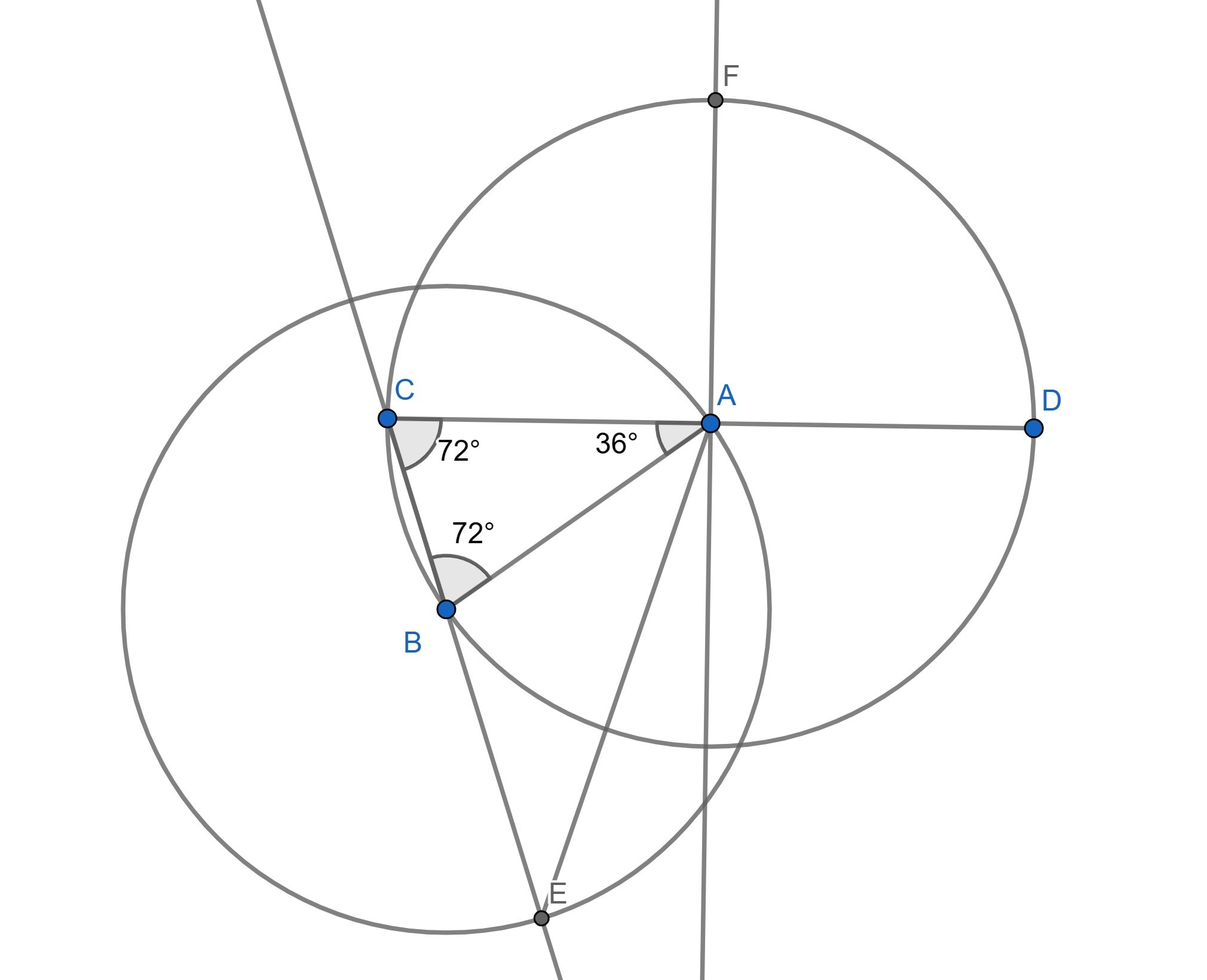
If CB is the side of a regular decagon, the angles at A, B and C will be as shown. Line CB is extended and a circle of radius AB centre B is drawn to intersect the line through CB at E. EA is joined. Since EBA is an isosceles triangle, angle E and the second angle at A will both be ##36^{\circ}.## It follows that triangles ECA and ABC are similar from which we may write the ratio equation: $$\frac{EC}{AB}=\frac{AC}{BC}\implies EC.BC=AB.AC={EB}^2 \implies \frac{EC}{EB}=\frac{EB}{BC}$$ showing that CE has been divided in mean and extreme at B with EB (radius or side of the hexagon) being the greater part and BC (side of decagon) the lesser.
The final piece of the ‘jigsaw puzzle’ to put in place (before we proceed with construction of a regular pentagon and decagon (or at least one side thereof) and subsequent calculation of side lengths) is the statement that the square on the side of an inscribed regular pentagon is the sum of the squares on the sides of the regular hexagon and decagon. Clearly, this is a Pythagorean relationship but invoking Pythagoras in an attempted proof only leads us back to the original statement. Copernicus directs us instead to Euclid XIII, 10 which reads as follows:
If an equilateral pentagon is inscribed in a circle, then the square on the side of the pentagon equals the sum of the squares on the sides of the hexagon and the decagon inscribed in the same circle.
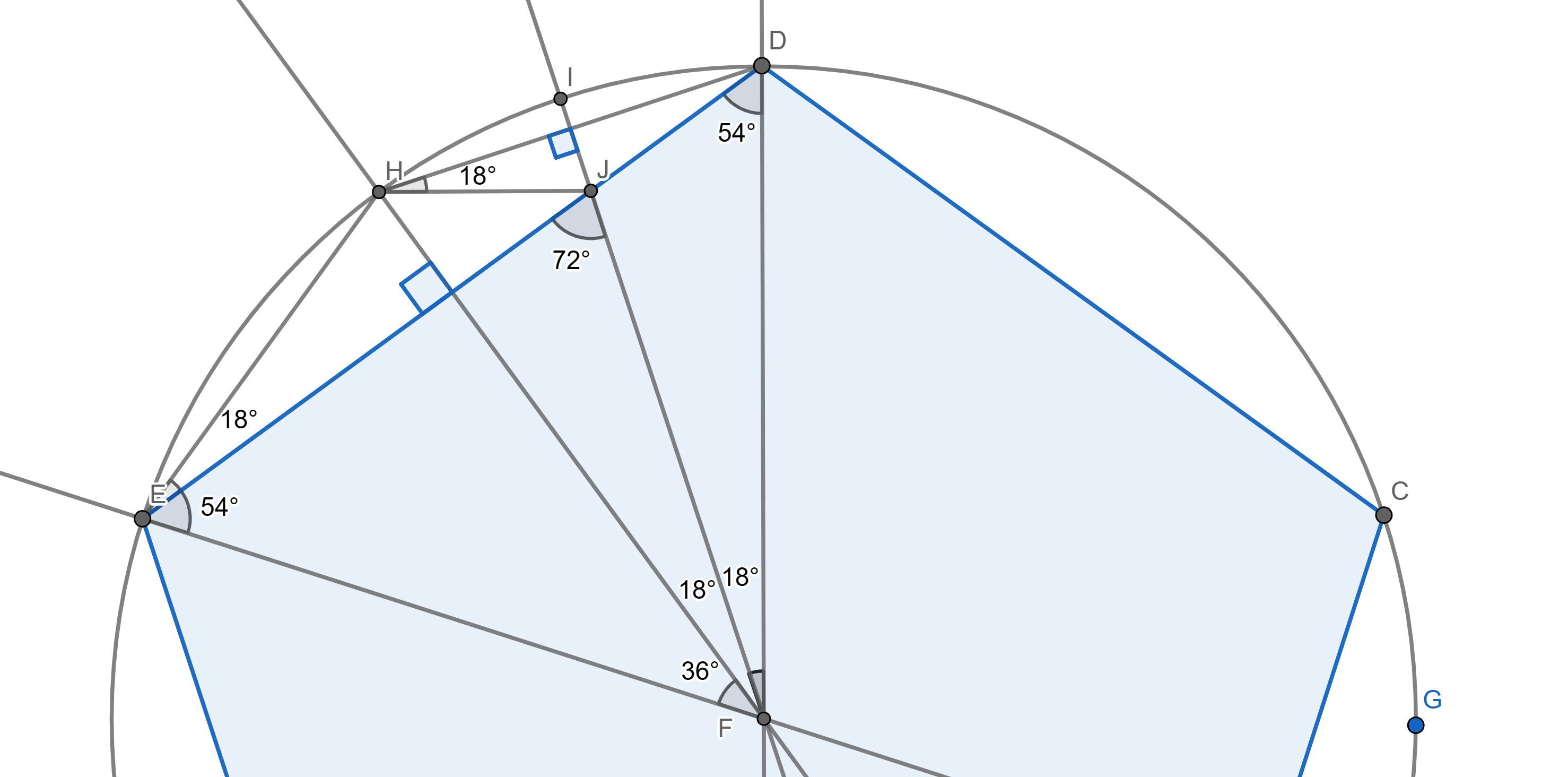
In the above diagram, ED is the side of a regular pentagon and EH=HD are sides of a regular decagon. F is the centre of the inscribing circle and lines FH and FI are drawn bisecting (respectively) side ED of the regular pentagon and side HD of the regular decagon. Angles are marked as shown and should need no commentary other than to note that the 72-degree angle marked at J is the external angle of triangle FJD and hence is the sum of angles JFD and JDF. Triangles HDJ and EDH are equiangular and therefore similar as are triangles EFJ and EDF. Hence we can write down the following ratio equations: $$\frac{HD}{ED}=\frac{DJ}{DH} \implies {DH}^2=ED.DJ\;…Eqn\;1.$$ $$\frac{EF}{ED}=\frac{EJ}{EF} \implies {EF}^2=ED.EJ \;…Eqn\;2.$$Adding Eqn 1 and Eqn 2 we obtain: $${DH}^2+{EF}^2=ED.DJ + ED.EJ=ED(DJ+EJ)={ED}^2.$$ But DH is the side of the regular decagon, EF the side of the regular hexagon and finally ED the side of the regular pentagon. So we have proved the statement given in Euclid XIII,10.
We may now proceed with the construction and calculation of the sides of the inscribed regular decagon and pentagon.

In the diagram above if we take the circle to be of unit radius, point G is a midpoint of radius AC. GB is joined so that by Pythagoras thm, GB is ##\frac{\sqrt{5}}{2}##. GH is constructed such that GB is equal to GH and therefore ##AH=\frac{\sqrt{5}-1}{2}=\phi##. AH is therefore the side of the inscribed decagon and we transfer this length to CL where C and L are points on the circle circumference. Half of chord CL is CM which corresponds to the sine of 18 degrees – ie ##\frac{\phi}{2} \approx 0.30902.## On the Copernican circle where the ‘unit’ radius is 100000 units, the corresponding table entry against ##18^{\circ}## will be 30902.
Radius BA is the side of the inscribed hexagon and AH is the side of the inscribed decagon. Remembering that the square on the side of the pentagon equals the sum of the squares on the sides of the hexagon and the decagon, BH will be the side of the inscribed pentagon. Length BH is therefore transferred to CI where C and I are points on the circumference and chord CI is the side of the inscribed pentagon subtended by ##72^{\circ}## at the centre. Half of chord CI is CJ which corresponds to the sine of ##36^{\circ}##. But chord CI is of length ##\sqrt{\phi^2+1}=\sqrt{1-\phi+1}=\sqrt{2-\phi}.## Hence ##\sin 36^{\circ}=\frac{\sqrt{2-\phi}}{2} \approx 0.58779## and the Copernican table shows 58779 units against ##36^{\circ}##.
Finally we may call upon the “Porism” referred to by Copernicus. If the circle is of unit radius then chord DI may be calculated as ##DI^2=CD^2-CI^2=4-(2-\phi)=2+\phi.## Now we resort to the extraordinary properties of the number ##\phi:## $$2+\phi=1+1+\phi=1+\Phi=\Phi^2.$$ Hence $$DI=\Phi=\phi^{-1}.$$ In triangle CID we have ##\sin54^{\circ}=\frac{DI}{CD}=\frac{\Phi}{2}=\frac{1}{2\phi}##. In the Copernican table where the ‘unit’ circle has diameter 200000 units, the entry against ##54^{\circ}## will be half of 161803 or 80902 units. Chord DL may be similarly calculated as ##DL^2=CD^2-CL^2=4-\phi^2=4-(1-\phi)=3+\phi.##. Hence ##DL=\sqrt{3+\phi}## and ##\sin 72^{\circ}=\frac{DL}{CD}=\frac{\sqrt{3+\phi}}{2}##. The corresponding entry against ##72^{\circ}## in the table of half chords will be half of 190211 or 95106 units.
Table of “Special Angles”
The following table summarises the quantities determined above.
| Radians | Degrees | Side of | Sine Ratio | Halves of chords subt. twice the arc |
|---|---|---|---|---|
| ##\frac{\pi}{10}## | ##18^{\circ} ## | Decagon | ##\frac{\phi}{2}## | 30902 |
| ##\frac{\pi}{6}## | ##30^{\circ} ## | Hexagon | ##\frac{1}{2}## | 50000 |
| ##\frac{\pi}{5}## | ##36^{\circ} ## | Pentagon | ##\frac{\sqrt{2-\phi}}{2}## | 58779 |
| ##\frac{\pi}{4}## | ##45^{\circ} ## | Tetragon | ##\frac{\sqrt{2}}{2}## | 70711 |
| ##\frac{3\pi}{10}## | ##54^{\circ} ## | ——– | ##\frac{\Phi}{2}=\frac{\phi^{-1}}{2}## | 80902 |
| ##\frac{\pi}{3}## | ##60^{\circ} ## | Triangle | ##\frac{\sqrt{3}}{2}## | 86602(3) |
| ##\frac{2\pi}{5}## | ##72^{\circ} ## | ——– | ##\frac{\sqrt{3+\phi}}{2}## | 95105(6) |
| ##\frac{\pi}{2}## | ##90^{\circ} ## | ——– | ##\frac{2}{2}## | 100000 |
In the last column bracketed digits indicate a marginal rounding error in the Copernican table and the bracketed digits indicate the correction to the final digit.
Summary and Conclusion

Therefore the diameter of the circle being given, the sides of the triangle, tetragon, pentagon, hexagon and decagon, which may be inscribed in the same circle, have been given – as was to be shown.
Acknowledgements
I would like to thank PF user Kuruman who kindly agreed to look over the article before I posted it for review here. It’s always encouraging to have a further “thumbs up” from one or other of PFs many experts so many thanks to PF user jim mcnamara who took time out to read the article and react in such a manner.
References
| [1] | Nicolaus Copernicus. De revolutionibus orbium coelestium: Liber Primus https://ads.harvard.edu/books/1543droc.book/, 1543. (Accessed on 11/26/2021). [ bib ] |
| [2] | Euclid et al. Euclid’s elements, Table of Contents. http://aleph0.clarku.edu/~djoyce/elements/toc.html. (Accessed on 11/26/2021). [ bib ] |
- BSc (Elec Eng) University of Cape Town, HDE University of South Africa
- Maths and Science Tutor, Florida Park, Johannesburg
- Research areas (personal interest): Hydrogen / Hydrogen-like spectra. Historical Maths.
- Wikipdedia contributions: Ptolemy’s Theorem, Diophantus II.VIII, Continuous Repayment Mortgage








Leave a Reply
Want to join the discussion?Feel free to contribute!